

Triangles: GSP Tools
by
Sarah Major
Equilateral Triangle
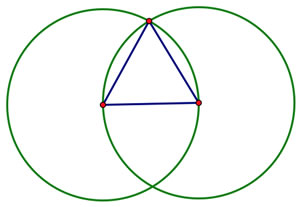
This triangle can be constructed be two equivalent circles. The second circle is formed by having the edge on the center of the first circle. This causes the overlap to be the radius of each circle. The other two sides are radii that result in a vertex where the two circles intersect. It does not matter if the vertex is the top or the bottom intersecton because they all are radii of the circles.
Isosceles Triangle
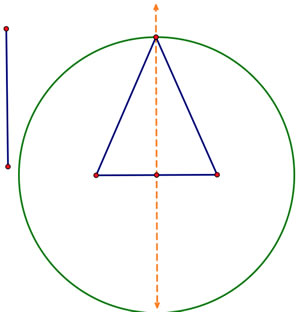
An isosceles triangle can be formed given an altitude an a base. Construct the midpoint of the base. Through this midpoint, construct a line. Select the midpoint and the altitude and construct a circle given the radius as the length of the altitude. Where the circle intersects the line is the length of the altitude from the midpoint of the base. Simply construct line segments from the endpoints of the base to the intersection point to complete the circle.
Medial Triangle
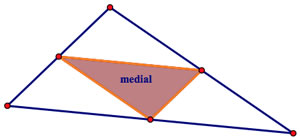
This is the triangle that results from finding the midpoints of each side of a triangle and then connecting them with segments. However, most people identify this triangle as the Triforce symbol from the Zelda video games:
Now we know how to save the Princess!
Orthic Triangle

To construct this triangle, construct the three altitudes of the triangle and find where they intersect. Then, connect the three intersection points with segments. Also see the Orthocenter.
Pedal Triangle

Construct any triangle and a point anywhere in the same plane. Then construct perpendicular lines from this point to each side of the triangle. The intersections of these lines create the vertices of the Pedal Triangle.