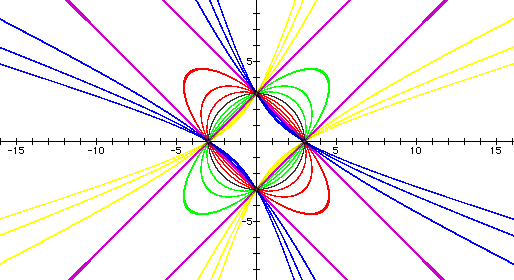
We will examine quadratic relations of the form for different values of n. In the following graphs, n = 0 (graphed in grey), n= 0.5, 1, and 1.5 (graphed in red), n = 2 (graphed in purple) and n = 2.5, 3 and 3.5 (graphed in blue).

When n = 0, the equation is the standard form of a circle centered at the origin with a radius of 3. When n = 0.5, 1 and 1.5, the relation graphs as a series of ellipses that become more eccentric as n increases. When n = 2, the left-hand side of the equation is a perfect square trinomial that factors into . Taking the square root of each side results in the equations for two parallel lines: x + y = 3 and x + y = -3. For values of n = 2.5, 3 and 3.5, the relation graphs as a series of hyperbolas.

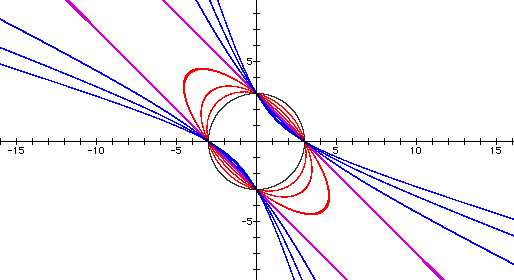
The next series of graphs demonstrate the effect of negative values for n in the relation. (The graph for n = 0 is included for comparison.)

Key: n = -0.5, -1 and -1.5, -2, -2.5, -3, and -3.5

The results for negative values of n are similar to the corresponding positive values of n. The obvious difference is the change in slope of each graph.

 

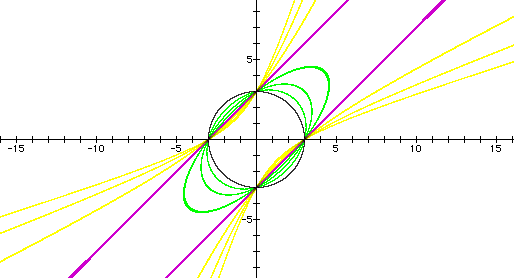
The following is an overlay of the previous two graphs: