

In this write-up, I examine the constructions of tangent circles for different cases. I will discuss the basic construction for the two primary cases, the loci of centers for the tangent circles constructed, the locus of a midpoint used in the construction of the tangent circle, and the construction of circles tangent to a circle and a line.
The task we are considering is the construction of a circle that is tangent to two given circles at a designated point. Consider the two circles below.
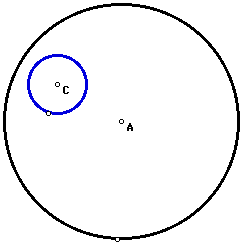
First we construct a point P on circle A and then construct the line through points A and P.

Next we construct a circle with center P and radius equal to the radius of circle C, and the point X where this circle intersects the line AP outside of circle A.

The segment XC is constructed, and then the perpendicular bisector of XC is constructed.
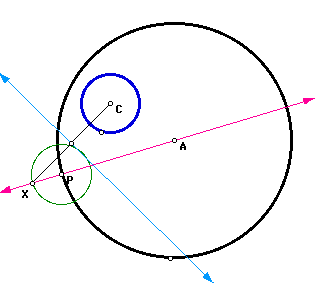
The center of the circle tangent to circle A and circle C at point P is the intersection of the perpendicular bisector of XC and the line AP.

Constructing a circle tangent to two circles so that the smaller circle is internal to the tangent circle is very similar to this construction. The only difference is that point X is chosen as the intersection of the circle with center P and the line AP that is inside circle A (versus outside circle A in the construction above). To use a GSP script to create a tangent circle externally tangent to two circles, click here. To use a GSP script to create a tangent circle internally tangent to two circles, click here.
When tangent circles are constructed, several cases can be considered. First we consider the cases where one circle is inside the other circle. For this situation, there are four separate cases. Case 1 is when the tangent circle is constructed external to both circles with the point of tangency on the larger circle. We have already seen the steps for constructing this tangent circle. The locus of the centers of these tangent circles can be seen by the envelope of the perpendicular bisector as the point of tangency is moved around the circle. The locus is an ellilpse with focal points at the centers of the original circles. To observe using GSP the envelope that forms the ellipse as the point P is moved around circle A, click here.
Case 2 is when the tangent circle is constructed with the smaller circle internal to the tangent circle and the point of tangency is on the larger circle. This case is shown below. To observe using GSP the envelope of the perpendicular bisectors as the point of tangency is moved around circle A, click here. The locus of the centers of the tangent circles is again an ellipse with foci at the centers of the original circles.
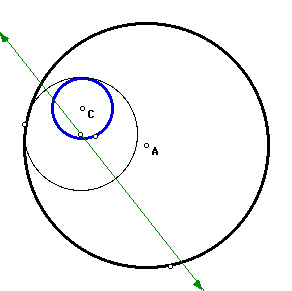
Case 3 occurs when the tangent circle is constructed external to both circles and the point of tangency is on the smaller circle.
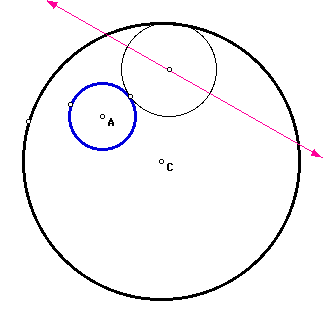
Case 4 occurs when the smaller circle is internal to the tangent circle and the point of tangency is on the smaller circle.
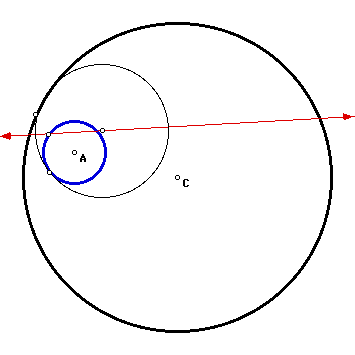
The loci of centers for all of the cases is an ellipse with focal points A and C, which are the centers of the original circles. To observe the envelope of the ellipse for Case 3 using GSP, click here. To observe the envelope of the ellipse for Case 4 using GSP, click here.
The next situation occurs when the two circles intersect each other. We will consider two cases, first when the point of tangency is on the larger circle and then when the point of tangency is on the larger circle. The locus of the centers for the tangent circles in these cases is also an ellipse with foci at the centers of the two original circles. Case 1 is shown below, and the envelope of the ellipse can be observed using GSP by clicking here.
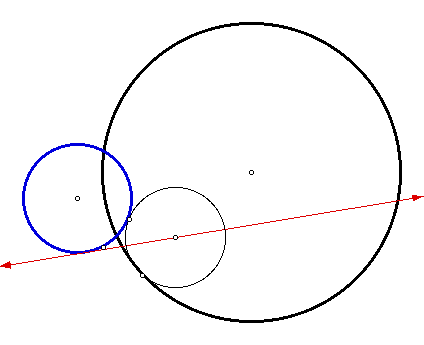
Case 2 looks very similar to case 1, and the envelope of the ellipse formed by the centers of the tangent circles can be seen in GSP by clicking here.

Next we consider the situation when the two circles are disjointed. There are four cases that occur in this situation. Case 1 exists when the tangent circle encloses either the larger circle or the smaller circle as the point of tangency is moved around the larger circle. To observe the envelope of the locus of centers for these tangent circles using GSP, click here. Case 2 exists when the tangent circle is either external to both circles or encloses both circles as the point of tangency is moved around the larger circle. To observe this case using GSP, click here. Case 3 occurs when the tangent circle encloses either the larger circle or the smaller circle as the point of tangency is moved around the smller circle. Click here to observe this case using GSP. Case 4 occurs when he tangent circle is either external to both circles or encloses both circles as the point of tangency is moved around the smaller circle. Click here to observe case 4 using GSP. An example of Case 4 is shown below. The locus of the centers of the tangent circles for all four cases is a hyperbola with focal points at the centers of the original circles.
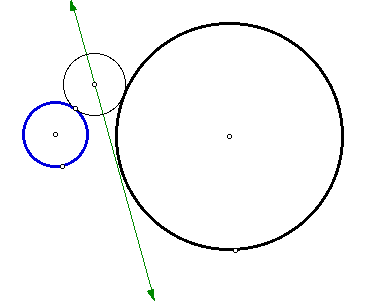
Students can use scripts to create these tangent circles and observe the changes in the loci of centers as the relationship between the two original circles changes. This observation shows that the locus of the centers appears to approach a parabola as the two original circles approach tangency. Students can use GSP to see that this locus is not a parabola, but instead a point at the center of the original circle on which the designated point of tangency is located. To observe that this is true, the students would need to construct two circles that are tangent to each other and then create a third circle tangent to both the original circles at a designated point. If the perpendicular bisector used in the construction of the third circle is traced as the point of tangency moves around one of the original circles, the trace will create an envelope around the center of the original circle on which the point of tangency is located.
Another interesting exploration is the locus of the midpoints through which the perpendicular bisector is formed when constructing a circle tangent to two given circles. These loci are circles whose center is at the center of the conic section formed by the locus of centers of the tangent circle constructed. The radii of the circles is half the fixed distance of the same conic section. For the cases when the loci of centers are ellipses, the loci of the midpoints are circles whose center is at the center of the ellipse and with radii of a length that is half the constant sum of the distances from the foci of the ellipse. To see an example of this situation animated in GSP for two circles that intersect, click here. For the cases when the loci of centers are hyperbolas, the loci of the midpoints are circles whose center is at the center of the hyperbola and with radii of a length that is half the constant difference of distances from the foci of the hyperbola. To see an example of the situation animated in GSP, click here.
Once students have learned to construct circles tangent to two given circles and then explored the different loci of points created during this construction for different initial cases, an extension of this theme would be the construction of circles that are tangent to a given line and a given circle at a point designated on the circle. The loci of the centers of the two tangent circles that can be constructed are parabolas. An example of this construction is shown below and an animation of the loci of centers using GSP can be seen by clicking here.

It would be a useful exercise for the students to explore this construction using GSP. To see an outline of this construction, click here.