
Tangent Circles

This investigation on tangent circles has many different problems associated with it. I will attempt to tackle a few of these ideas within this write-up but I encourage you to try to solve a few others (or expand on mine) ideas about this concept. To see a list of such problems go to:
For this investigation, GSP 4.0 will be put into use. We will use GSP to find tangent circles for two cases.
CASE I - Circle in the interior of another circle.
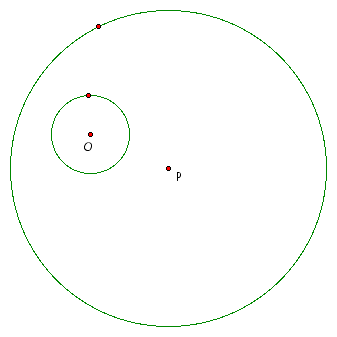
We want to construct a circle that is both tangent to circle P and circle O. The first step is to pick an arbitrary point on P, (we will label this A) and run line through P and A. The resulting sketch will be so:

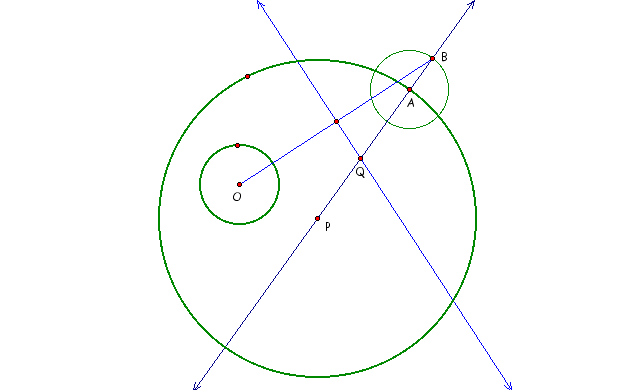
We want to construct a circle that is tangent to P at A and tangent to circle O. The center of such a tangent circle will lie on line PA. With a bit of investigation it should be clear that the center of the tangent circle will be between points A and P. The question is how can we find this center? The key to this is to construct a congruent circle to O with a center at A. After doing this the sketch will change to this.

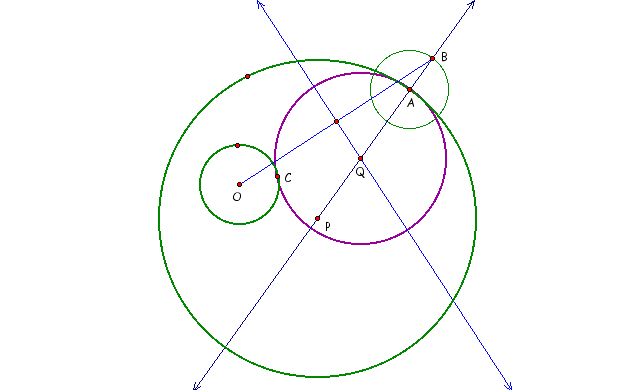
Now the center of our tangent circle will be equal distance from points O and B. To find the tangent circle we must use the idea of a isoceles triangle to construct the point. So the base of the isoceles triangle (OB) will be constructed then the midpoint of the base and the altitude of the triangle. The intersection of this altitude and line BP will be the center of our tangent circle.
Constructing a circle running through point A and having a center of Q we will have a tangent circle.
Proof that this construction is correct: CLICK HERE
With this construction the placement of A on circle P will always produce a circle the is tangent to circles O and P. If we move A to a different spot we can get this sketch:
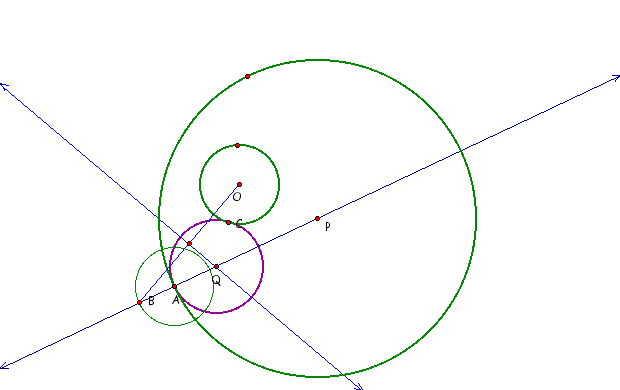
(For GSP 4.0 users) To see point A animated along circle P, CLICK HERE
As we have noticed there are an infinite number of these tangent circles based off this construction. Let's look at the locus of the centers of these circles:
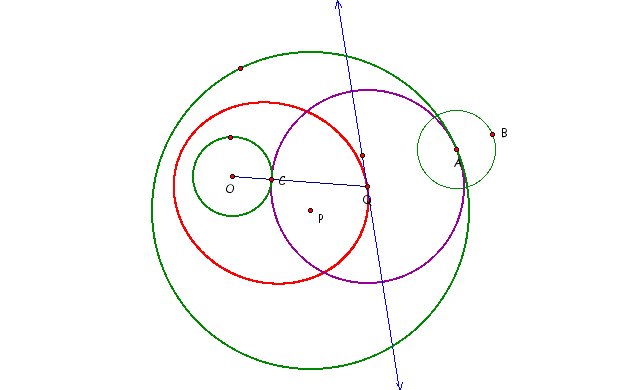
We notice that it seems as if we have an ellipse with foci at O and P. We notice that the line running through Q is a tangent line to the ellipse. If we look at the locus of these tangent lines we should get a sketch that envelopes the ellipse:

Upon inspection our idea was true. Now we have looked at one tangent circle for the given case, while in fact we can construct a second:
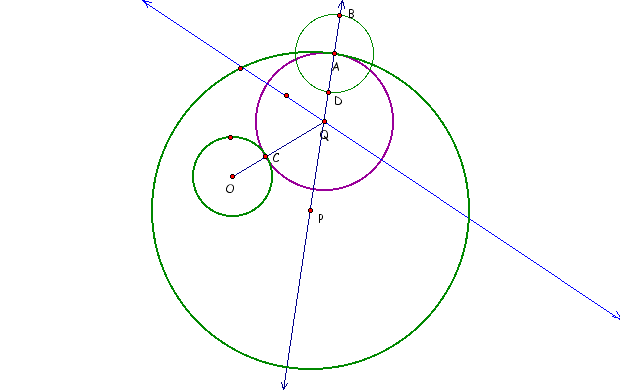
Notice the addition of point D that intersects circle A and line PA. The construction of this second tangent circle is quite similar to the first except we will run the base of our isoceles triangle from O to D to find the center on line PA. If we do this the construction will look as so:
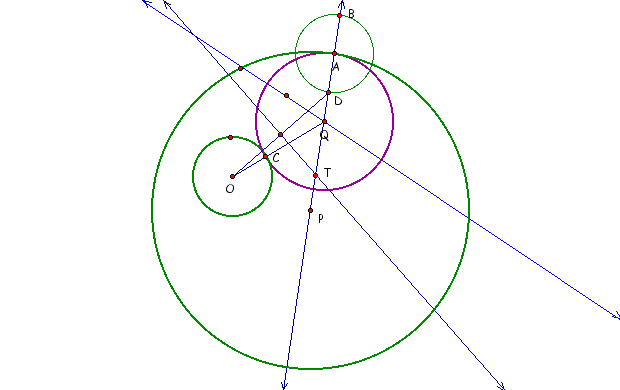
Clearly we found the altitude to the base of our second isoceles triangle and found the vertex at point T. Constructing a circle with center T and passing through A we will find our second tangent circle. The proof that this is in fact correct is quite similar to the earlier proof and will be left up to you to convince yourself. Our second tangent circle: (Notice we have now hidden some components)
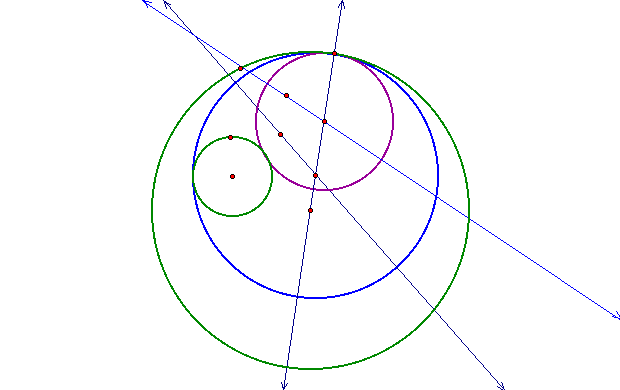
Clearly we have constructed two tangent circles that are both tangent to the large circle at an arbitrary point and tangent to the smaller circle at two different points. Earlier we looked at the locus of the centers of the first tangent circle and thought our locus was an ellipse. Now let's look at the locus of the centers of our second tangent circle:
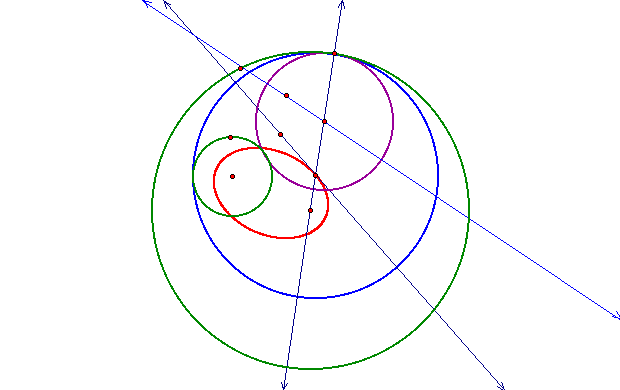
and the envelope of lines for this ellipse:
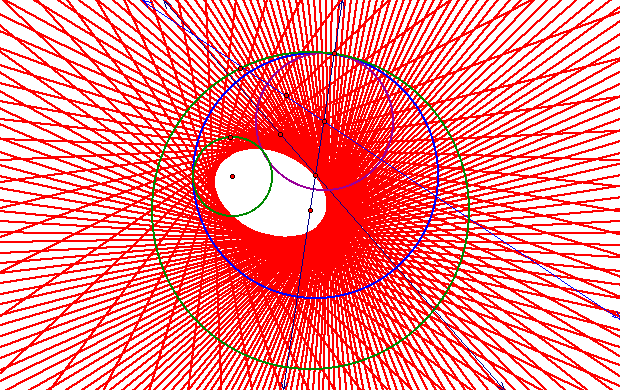
It seems as if the two ellipsis have the same foci let's put these two loci together from both tangent circles:
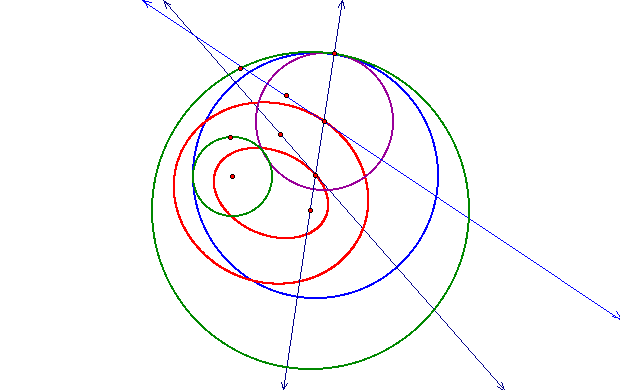
We have investigated tangent circles when the smaller is in the interior let's look when the smaller is outside of the larger:
CASE II - Smaller circle in the exterior of the larger circle.
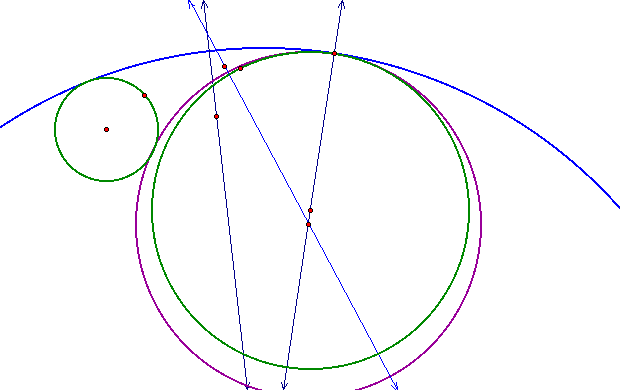
(GSP 4.0 Users) By moving the smaller circle out we see that the tangent circles are forced to increase in magnitude. To see the set of these two circles
If we look at the loci of the two centers of the circles we get these sketches:
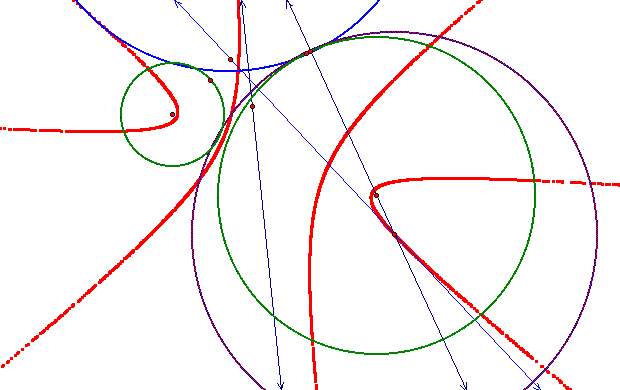
We know do not have a pair of ellipsis but in fact a pair of hyperbolas. Note GSP does not proof this but appearance seems to tell us this fact.
This investigation into tangent circles is just the small taste into the mathematics of this scenario. I would hope that the construction of such tangent circles would make better sense than they did earlier. Remember to see other questions/problems about this situation please go to the URL given at the beginning of the investigation.
RETURN to Brock F. Miller Page