Investigation of Quadratic Equations
of the Form y=ax2+bx+c
By: Denise Natasha Brewley
In this investigation I will explore three forms of y = ax2+bx+c. I will first vary 'a' and fix the other two terms. And do the same for the other terms 'b' and 'c'. Before we begin, we will discuss how to find the roots of the parabola of the form
y=ax2+bx+c
In order for us to find the roots of the equation, I will set y=0. So we obtain,
ax2+bx+c=0
and so it follows from the Quadratic Equation that the roots are
x=(-b ± Öb2-4ac)/2a
I will use this result for the three investigations.
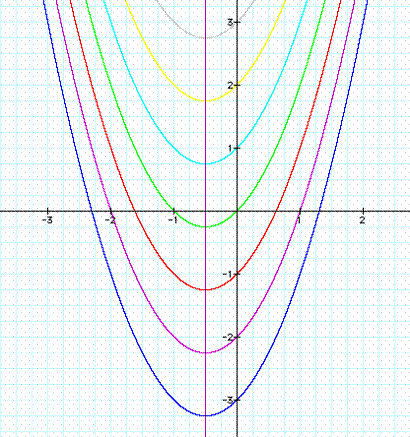
Investigation 1: Vary a, b=1 and c=1
I first begin this investigation by considering the quadratic equation of the form y = ax2+x+1.
|
|
|
|
|
I considered the range of 'a' when - 3 < a < 3. Notice in the case when a is not equal to zero, we obtain parabolas whose vertices can be found by x=-1/(2a). Also note that as the value of 'a' varies, the parabola either have a maximum or minimum at x=-1/(2a). If a > 0, then there are no real roots to the equation y = ax2+x+1. This follows since, x=(-b ± Ö(b2-4ac))/2a =(-1 ± Ö(12-4a(1)))/2(1). Since the discriminant is negative, no real roots. When a < 0, both negative and positive roots are obtained. This can be verified by using the previous result, x=(-b ± Öb2-4ac)/2a. In the case when a=0, we obtain a line y=x+1. It is interesting to note here that y=x+1 acts as an inflection line for the family of parabolas. Essentially, this is where the concavity of the parabolas change from concave up to concave down.
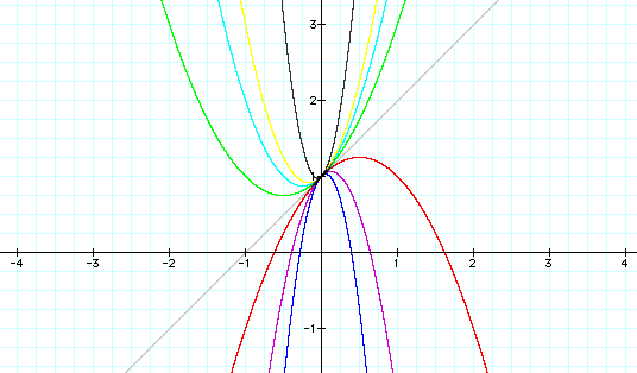
Investigation 2: Vary b, a=1 and c=1
Now let us look at the case when y = x2+bx+1.
|
|
|
|
|
To save time I have included the family of parabolas where -3 < b < 3, along with the parabola y = -x2+1. The equation y = -x2+1 represents the locus of the vertices of the set of parabolas of equation y = x2+bx+1. I will first discuss the roots of y = x2+bx+1 and then I will show that y = -x2+1 is the locus of vertices. I first considered the case when b < 0. Notice that the parabola has two roots that are both positive. This makes sense because the location of the vertex is x= -b/(2a). It follows that x = b/(2). And so the roots of parabolas in this case are x=(-b ± Ö(b2-4ac))/2a = x=(-b ± Ö(b2-4(1)(1)))/2(1). If is important to mention here that if b2< -4ac, then there are no real roots. We can apply this same reasoning for the case when b > 0. In this case, the location of the vertex is x=-b/(2a) and it follows that x = -b/(2). Also note here that if b2 >-4ac, then there are real distinct roots.
Now as for y = -x2+1, let us first consider the equation y=ax2+bx+c. I will take the derivative of and it follows that
y' =2ax+b
We now want to set the derivative equal to zero, and so
2ax+b=0
Solving for 'x' we obtain,
x=-b/2
Substituting this value of 'x' into y = -x2+1 yields
y = -(-b/2)2+1
which simplifies to
y = -(b2/4)+1
And so for any value of 'b' that is chosen, the point (b, -(b2/4)+1) will be on y = -x2+1.
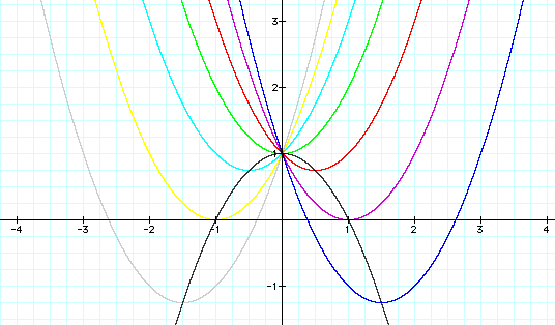
Investigation 3: Vary c, a=1 and b=1
The final case in this investigation is when y = x2+x+c.
|
|
|
|
|
In the last case, I also looked at values of 'c' in the range -3 < c < 3. As you can see, the family of parabolas for y= x2+x+c have vertical translations. Each value of 'c' defines a y-intercept for its respective graph. I will now proceed with the same discussion of the the roots as in the last two cases. Again, consider x=(-b ± Ö(b2-4ac))/2a. When c > 0, we get x=(-1 ± Ö(12-4(1)c)))/2(1), no real roots are obtained. When c < 0, we get x=(-1 ± Ö(12-4(1)c)))/2(1) which gives us real roots.
|
|
|
|
|
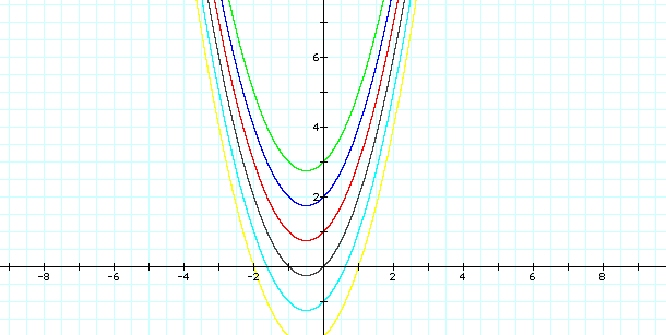
To conclude this investigation, I wanted to know what the locus of the vertices of the set of parabolas would give us. What we end up with is a vertical line through the value x=-1/2. So let us prove it. From above, we saw that the derivative of our general quadratic was y' =2ax+b. It followed that 2ax+b=0 and x=-b/2. We now set x=-b/2=-1/2 and b=1, which was given. And so for any value of 'c' that is chosen, the point (-1/2,(-1/2)2+(-1/2)+c)=(-1/2,-1/4+c) will be on x=-1/2.