Assignment 6
Problem 1
Relationships
between a Triangle and its Medial Triangle
by
Kenneth
E. Montgomery
Problem
Construct a triangle and its medians. Construct a
second triangle with the three sides having the lengths of the three medians
from your first triangle. Find some relationship between the two triangles.
(E.g., are they congruent? similar? Have same area? same perimeter? ratio of
areas? ratio or perimeters?) Prove whatever you find.
Overview
Relationships between the segments and angles formed
in the construction of a triangle and its medial triangle are investigated.
This problem was explored using the Key Curriculum Press, Geometer’s Sketchpad
® software. Questions regarding the ratio of areas and perimeters as well as
similarity and congruency of angles, sides and triangles are explored using the
software. Observations are made and hypotheses tested, within the exploration,
and a proof of each result is offered below.
Investigation
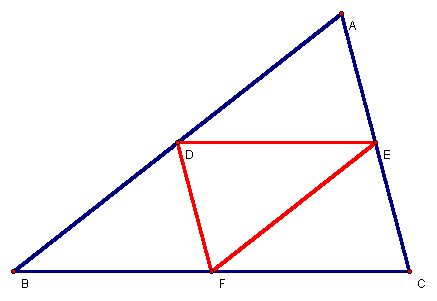
Upon construction of 
,
the midpoints (D, E and F) as are
the connecting segments, 
and 
,
(Figure 6.1). It should be noted that individual segments are also constructed
between points A and D, et cetera, so that measurements of each individual
segment within the triangle may be found.
with
medial 
|
|
|
|
|
|
|
|
|
|
|
|
Table 6.1
From these measurements it is clear that, for this
specific triangle at least, congruence relationships exist. Further, we
hypothesize congruence relationships for angles. To investigate, it is
necessary only to select three points, which together represent an angle
(e.g. D, B and F for 
). Once these points are selected, we
select Angle from
the Measure menu. The
hypotheses should be verified, again for this triangle in particular, by
observation of the measurements in Table 6.2.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Table 6.2
An understanding of congruent triangles should be
developed from this exploration. We may at this time conjecture that 
. This investigation helps to distill an
argument for why the original triangle (
) is not congruent to the medial triangle (
). Further investigation of segment length in 
produces the data in Table 6.3. A comparison of
these values to those in Table 6.1 provide a clear argument as to why the two
triangles are not congruent: corresponding side lengths are not congruent.
|
|
|
|
Table 6.3
What we notice, however is that the ratio of side
lengths is 2:1 and this is also verified in Sketchpad® by selecting two
corresponding segments, such as 
and 
and selecting Ratio from the Measure menu. The
ratio is verified for all three pairs of angles. We conjecture that the
triangles are similar and by any of the three theorems for similarity (SSS,
SAS, ASA) we can empirically verify that they are.
The question that arises is whether any of our
hypotheses are true in general, or just for this triangle. Again, Sketchpad®
may be used to investigate, by either constructing different triangles (and
repeating our previous steps) or by dynamically changing the properties of our
original triangle, while measuring each of the quantities mentioned. A GSP file is provided as an illustration. However, while
we have verified the above-mentioned properties for more triangles than just
our original, we have not yet proven this in general, nor have we addressed the
issues of perimeter or area.
Note to self: come back and fix
fonts.
Proof:
Given 
, with constructed midpoints and segments, we
have the medial triangle, 
(Figure 1). Since D is the midpoint of AB, F is
the midpoint of BC and E is the midpoint of AC. It follows that AD=DB, BF=FC,
and BE=EC.
By the SSS Theorem (Side, Side, Side), we have 
. By the CPCTC Theorem (Corresponding Parts of
Congruent Theorems are Congruent) we also have congruent angles: 
and 
as well as
.
The Definition of Similar Polygons states that two
polygons are similar if and only if their corresponding angles are congruent
and the measures of their corresponding sides are proportional. Therefore,
since
Proof:
Part 1: 
, with constructed midpoints and segments, we
have the medial triangle, 
(Figure 1). Since 
, 
and 
, it follows that 
, 
and 
.
Since 
and
, we have
and 
. By the reflexive property, we have
and 
by SAS similarity. Ò
Part 2: 
, we have 
and without loss of generality, we also have
and
. Thus
and
, with
(reflexive property). By SSS congruence we thus
have
.Ò
Part 3: 
) is composed of the smaller triangles, we have:
