Assignment #8
Nicole Mosteller
EMAT 6680

Altitudes and Orthocenters
The first interesting property in this investigation of altitudes and orthocenters occurs when finding the orthocenter
of the triangle whose vertices are two vertices of the original triangle and the orthocenter of the original triangle.

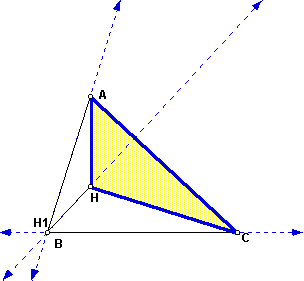Figure 1:  H is the orthocenter of the original DABC.
The orthocenter for DACH has label H1 and is point B.

Once we recall how point H is located, it will be clear why H1 and B are the same points.

H is on the line (BH) containing B that is perpendicular to segment AC,
H is on the line (AH) containing A that is perpendicular to segment BC, and
H is on the line (CH) containing C that is perpendicular to segment AB.

To find the orthocenter of DACH, notice that the perpendiculars to the sides AH and CH 
are sides of DABC (sides AB and CB).  These segments clearly intersect at point B.
Recall that the line perpendicular to side AC through H contains point B.
B is the orthocenter of DACH.

Why are the circumcircles of the original DABC, DABH, DBCH, and DACH congruent? 


Return to Nicole's Page