Check Problems from last lesson
Review: Write in your Journal all that you remember from the beginning of the lesson. Don't go back yet to check. Do from memory.
Check
your memory, noting what you need more work on.
Activity: The Isosceles (45-45) Right Triangle. An Isosceles right triangle is a special triangle with several special properties. Thes properties result in shortcuts that make it easy to find unknown measures of parts of an isosceles right triangles
Click here and print the GSP activity.
Activity: The 30-60-90 Right Triangle. The right triangle, which is half of an equilateral (equiangular) triangle, has special properties also.
Click here and print the GSP activity
Isosceles
Right (
)Triangle Theorem: In
an isosceles right (
) triangle, the
hypotenuse is 
times as long as
a leg.
Triangle Theorem:
In a 
triangle,
the hypotenuse is twice as long as the shorter leg and the longer
leg is 
times as long as the shorter leg.
In the triangle to the right we
can apply the Pythagorean thereom to the hypotenuse, s :
So we see that the sides in a 
triangle
are 
.
Examples of using the Isosceles Right
Triangle and 
Theorem:
Find x:
1.
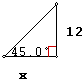Since we have an isosceles right
() triangle, the legs are equal, so
x =12.
2. 
Since we have an isosceles right
()
triangle, the hypotenuse is as long
as the leg so
x = 12.
Find the Area of the following triangle:
We are given the length of the hypotenuse so we will first
use this part of the 
Theorem
which states: the hypotenuse is twice the shorter leg, so the
shorter leg (the base of our triangle) is half the
hypotenuse,or, 
feet.
The next part of the theorem says longer leg is times
as long as the shorter leg. So the altitude of our triangle (the
longer leg) is 
feet. The formula
for the area of a triangle is 
base
times altitude, so we can find the area of our triangle is :
feet.
Click the lightbulb to practice what you've learned.
