

by Robyn Bryant, Kaycie Maddox, and Kelli Nipper
The following assignment is a possible investigation for high school students. It involves using GSP to investigate quadrangles. We will state the problem and then write an essay discussing our own observations of the problem. This is not written in a form that a student could just pull up and work from. It is more designed for a teacher to use as a guideline for a classroom assignment.
Student Prerequisites: Students must be familiar with using GSP, writing proofs, and the following vocabulary - points, segments, collinear, vertices, and intersect.
Definition: A quadrangle is a collection of four points A,B,C,D (the vertices of the quadrangle), with four segments AB,BC,CD, and DA (the sides of the quadrangle), such that no three of the vertices are collinear. (The sides AB and CD may intersect or the sides AD and BC may intersect.)
A. What kind of figure is formed when the midpoints of the sides of a quadrangle are joined in order?
B. What is the relation of the area of this figure to the area of the quadrangle?
C. Once you have investigated A and B, then you must make a conjecture regarding each one and then write a proof of your theories.
Part A.
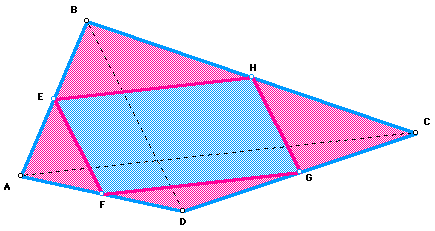
The following is a picture of a quadrangle with the midpoints of the segments joined. To manipulate this picture, click here.

Conjecture: The figure formed when the midpoints of the sides of a quadrangle are joined in order is a parallelogram.
I began by creating what was given. The first thing I did was to investigate what kind of figure was formed when the midpoints of the sides of a quadrangle are joined in order. It appeared to be a parallelogram, but was it so in every case? I moved vertex D around to see if the polygon created still appeared to be a parallelogram. It did. I also moved D so that CD would cross AB. Even then the shape appeared to be a parallelogram. The only time a parallelogram did not exist was when the diagonals of the quadrangle were parallel. To investigate this situation, click here.
Proof:

In triangle ABD, EF is parallel to BD, because segment EF bisects two sides of the triangle. In the same way HG is parallel to BD in triangle BCD; EH is parallel to AC in ABC; and FC is parallel to AC in ACD. Since we have 2 pairs of parallel lines (EH ll FG and EF ll HG) we have a parallelogram, by definition.
Part B.
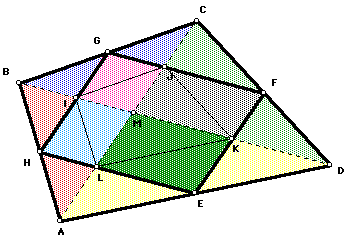
The following is a picture of a parallelogram formed inside a quadrangle by joining the midpoints of the segments of the quadrangle. To manipulate the quadrangle and observe the area of the two figures, click here.
![]()
By looking at GSP and moving a vertex of the quadrangle around, i have come to a conjecture.
Conjecture: The area of the constructed parallelogram is half the area of the quadrangle.
I did not have an idea of the relationship between the two areas until I chose to measure the areas of the two and then calculate the ratio. Once I had the ratio, I moved point D around and noticed that the area of the parallelogram was always half the area of the quadrangle. Now the question is "Why?" That's when I decided to divide the figures up into triangles and compare their areas with one another.

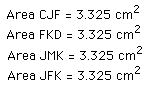
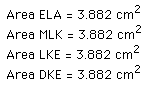
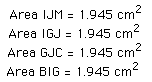
Proof:
We have already proven that FJ, DB and HE are all parallel to each other and so are FE, AC, and HG. So let's look at parallelogram HLMI.
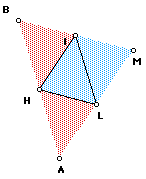
The diagonal IL forms two triangles IHL and IML. The area of these two triangles are congruent by the ASA theorem. Let's now look at the area of triangles BIH and ALH. By using corresponding angles, we know that <HAL is congruent to <BHI and <BIH is congruent to <HLA. Since H is the midpoint of AB, we also know that BH is congruent to HA, therefore, by AAS, triangle BIH is congruent to ALH.
Using alternate interior angles we can say that <BHI is congruent to <HIL and that <BIH is congruent to <LHI. By ASA, triangle BIH is congruent to ALH. By substitution, we can now say that all four triangles are congruent. Now looking at the whole triangle BMA, which is the total area of the four congruent triangles, is twice the area of the parallelogram IHLM.