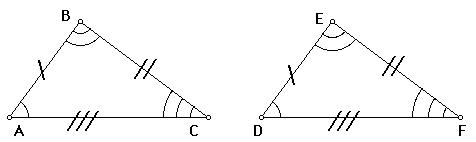
Objective: (same as Day 3)
GA QCC: #16
Lesson: Triangle Congruence (SSS, SAS)
NAME: ________________________
1. From the last lesson, we said that if 2 triangles are congruent, then the corresponding angles and corresponding sides are congruent. If we say it another way, 6 pieces of information from one triangle is congruent to the corresponding 6 pieces of information to the other triangle.
2. The question is, "Do we need to know all 6 pieces of information on each triangle to conclude that the 2 triangles are congruent?" The answer is "No."
3. There are 2 postulates (statements that are given without proofs) that can be used to prove 2 triangles are congruent to each other without using all 6 pieces of information per triangle:
SSS: Side-Side-Side Triangle Congruence Postulate
SAS: Side-Angle-Side Triangle Congruence Postulate
(which refers to side, included angle, and side of one triangle...)
4. What both of these postulate say is that if we know these pieces of information (SSS, for example) of one triangle being congruent to the same information (ie, corresponding parts) of another triangle, then the 2 triangles are congruent. The same statement can be made for a SAS situation. As an example, the following triangles are congruent because of the SAS Congruence Postulate.

5. Click HERE (Figure 3) to answer the following questions. To prove the triangles are congruent, state which postulate you would use (SSS or SAS), both, or neither.
5a. ________________________ 5b. __________________________
5c. ________________________ 5d.___________________________
5e. ________________________ 5f. ___________________________