Unit 5 - Getting From One Orbit To Another

Discussion

We began this whole series of lessons considering the issue of the Mars Pathfinder getting from earth to Mars, and while we may not have stated it as clearly, we really characterized that problem as one of getting from an object in one orbit to an object in another orbit by means of a third orbit (the orbit of the Pathfinder) - that is we were really interested in the intersections of the orbits and more particularly in being sure that Mars would be waiting at the second intersection at the critical moment.

The challenge of getting the Pathfinder from Earth to Mars can also be thought (and this is probably more realistic) as a transfer of orbits. That is the Pathfinder is really traveling in the Earth's orbit as long as it is sitting on Earth, when it reaches Mars it will, by the same token, be traveling on Mars' orbit. This change of orbit idea is an important part of space flight for other purposes as well.

When shuttles launch satellites and similar vehicles they literally release them at some critical moment. Unless some force, such as a thrust by the satellite's rockets, acts on the satellite it will have the same initial velocity as the shuttle that released it and this velocity, the mass of the satellite and its distance from the Earth will now determine its unique orbit (Kepler's and Newton's laws). However, depending on the role that the satellite is to play it may need to go to a new orbit. This change of orbit is achieved by the strategic use of the satellite's thruster rockets. While there are many different ways in which the navigators can achieve this (recall Pieter Kallemeyn's journal discussions) one of the most economical and simple transfers is called a Hohmann transfer.

Hohmann Transfer Orbits

Some important general remarks:

• There are essentially two kinds of "burns" (or thrusts) that space vehicles use:
  • posigrade burn is a burn that increases the forward speed of the vehicle,
  • retrograde burn is a burn that decreases the forward speed of the vehicle.
• An increase in forward speed of the vehicle will place it in a "larger" orbit.
• A decrease in forward speed of the vehicle will place it in a "smaller" orbit.
• If a vehicle in some orbit experiences a burn and changes orbit, if no other burn takes place then the new orbit will intersect the old orbit at the point of burn.
• The orbit transfers that we are dealing with here are restricted to in-plane transfers, i.e. the old and new orbits are in the same plane - this suits us well given the simplifications we are working with.

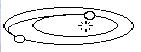
To understand Hohmann Transfer Orbit consider the diagram alongside. A vehicle is traveling in some orbit A around the Earth and we want to get it to orbit C. At some point the engine performs a posigrade burn thus enlarging the orbit and the vehicle is now traveling along orbit B. The point where the posigrade burn took place becomes the point of perigee of the new orbit (B). Unless there is a further burn the vehicle will now continue to move in orbit B.

Since we want to move the vehicle to orbit C, the size of the posigrade burn (at perigee) was well designed to ensure that the point of apogee (of orbit B) meets orbit C. At apogee a further posigrade burn is used to enlarge the orbit again this time the vehicle goes into orbit C and the transfer is complete.

The diagram is also an excellent illustration of how Pathfinder moved from Earth to Mars. In the case of Pathfinder the Sun was at the center, A represented the orbit of the Earth, C the orbit of Mars and the perigee and apogee points are called the perihelion and aphelion respectively because the orbits are Sun-centered. It is interesting to note that launch opportunities that ensure the correct positions of Earth and Mars for the success of this transfer only occur every 25 months (imagine all the good fortune that allowed a landing on July 4, American Independence Day).

Finally consider the case of C being the orbit of the Earth and A the orbit of Venus about the Sun. To send a vehicle from Earth to Venus would be like performing a Hohmann transfer from orbit C to orbit A, this time only we would use a retrograde burn at the apogee/aphelion point of B to transfer the vehicle to orbit B and a further retrograde burn at the perigee/perihelion of B to transfer the vehicle to A.

Click the button to read about other orbit transfers.

Student Tasks

Orbit	Perigee	Apogee
Stage 1	300 km.	375 km.
Stage 2	540 km.	7500 km.
Stage 3	15000 km.	27000 km.
Stage 4	540 km.	375 km.

• In this task you are given some data for the orbits of four subsequent stages of some imagined space mission, your task is as follows for each of the following pairs of orbits (stage 1 and stage 2), (stage 2 and stage 3), (stage 3 and stage 4) and (stage 4 and stage 5):
  • Plot both orbits, and
  • Plot a Hohmann transfer orbit that will take you from the first orbit in the pair to the second.
  • You can click here for a look at my solution for the stage 2 to stage 3 transfer.
• If your graphing utility will allow it try make the following graph for each pair:
  • Let the vehicle spend one revolution in the first orbit, then the minimum time in the Hohmann transfer orbit and finally one revolution in the second orbit.
• Write a short paragraph describing the transfer for one of the pairs, i.e. describe the transfer in terms of the kinds of burns and when they are used.
• CHALLENGE: try to use your graphing utility to do the following:
  • let the vehicle spend one revolution in orbit 1 and then move to orbit 2, spend one revolution in orbit 2 and then move to orbit 3, spend one revolution in 3, move to 4 spend one revolution in 4 and finally return to orbit 1.
• BIG CHALLENGE try to find the various orbital elements for the planets and design some Hohmann transfer orbits to take you from one planet to another (more accurately from the orbit of one planet to the orbit of another - since we still do not have any way of dealing with the time issue). Remember that this problem will involve Sun-centered orbits!