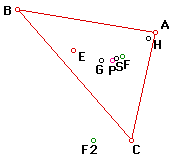

Now E is the point of concurrency of the of the lines joining the centroids
of the internally constructed equilateral triangles and the vertices of
the original triangle.

E, as is to be expected, is a point on the locus of D. However, what
is more interesting is that E is a member of the same branch of the hyperbola
as the other points that have positive base angles (see construction description
Task D). This raises the interesting question for
what values of the base angle will the point D be a member of each of the
two branches of the hyperbola?
From the work done we know that the one branch contains the following points:
H-- orthocenter: base angle = 90 deg
F -- Fermat point: base angle = 60 deg
S -- concurrency point for the centers of squares problem: base angle =
45 deg
P -- concurrency point for the centroids of equilateral triangle problem:
base angle = 30 deg
G-- centroid: base angle = 0 deg
E -- concurrency point for the centroids of internally constructed equilateral
triangle problem: base angle = -30 deg
While the other branch contains the point F2 -- second Fermat point: base
angle = -60 deg.
This question is left as a problem for the reader.
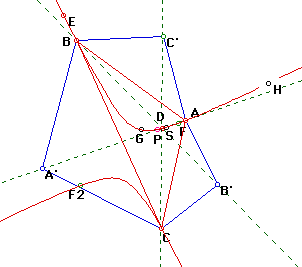
To investigate the problem suggested above and to watch a GSP drawing
of the locus click here.