
The Department of Mathematics Education

It has now become a rather standard exercise, with available technology, to
construct graphs to consider the equation
and to overlay several graphs of
for different values of a, b, or c as the other two are held constant. From
these graphs discussion of the patterns for the roots of
can be followed. For example, if we set
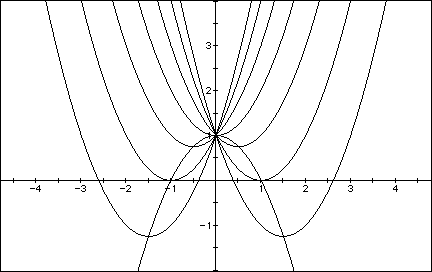
for b = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture
is obtained.
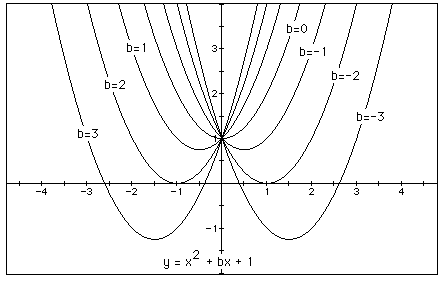
We can discuss the "movement" of a parabola as b is changed. The parabola
always passes through the same point on the y-axis ( the point (0,1) with this
equation). For b < -2 the parabola will intersect the x-axis in two points
with positive x values (i.e. the original equation will have two real roots,
both positive). For b = -2, the parabola is tangent to the x-axis and so the
original equation has one real and positive root at the point of tangency. For
-2 < b < 2, the parabola does not intersect the x-axis -- the original
equation has no real roots. Similarly for b = 2 the parabola is tangent to the
x-axis (one real negative root) and for b > 2, the parabola intersects the
x-axis twice to show two negative real roots for each b.
Now consider the
locus of the vertices of the set of parabolas graphed from
We know that the vertex of a parabola is the maximum or minimum point of the parabola. To find this point, we can take the derivative of the function
![]()
and set f'(x) = 0 to find the x-value of the vertex.
![]()
![]()
![]()
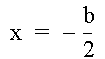
To find the y-value of the function at this point, we plug x = - b/a back into the original equation. We get
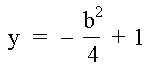
So we know that the point
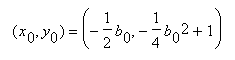
is the vertex of the parabola when b = b0. Thus, we need to find the parabola
![]()
that goes through the point (x0, y0). Plugging (x0, y0) into the above equation, we have
![]()
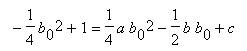
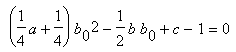
We now have a quadratic function in b0. Setting the coefficients of b02, b0 and 1 equal to 0, we see
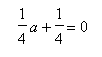
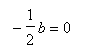
![]()
Therefore, a = -1, b = 0, and c = 1. So the locus of the vertices of the set of parabolas graphed from
is the parabola
Recall that the roots of the equation
![]()
are given by the quadratic formula. Thus, the roots of the equation are
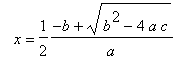
and
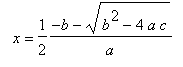
Real roots exists if and only if the determinant
![]()
is non-negative. There are two real roots if the determinant is positive, one real root if the determinant is zero, and zero real roots if the determinant is negative.
Consider the equation
![]()
When we graph this relation in the xa plane, we get the following graph.

If we take any particular value of a, say a = 1, and overlay this equation on the graph we add a line parallel to the x-axis. If it intersects the curve in the xa plane, the intersection points correspond to the roots of the original equation for that value of a. We have the following graph.
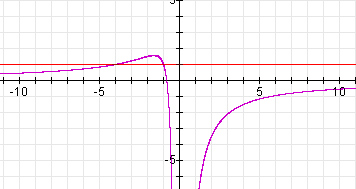
Thus, the roots of the original equation are -4 and -1.
For each value of a that we select, we get a horizontal line. It is clear on the above graph that we get two real roots when a < 1.5625, one real root when a = 1.5625, and no real roots when a > 1.5625.
From the above discussion about roots, we know that we should expect one real root when

If c is positive, we can expect two real roots when
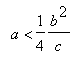
and no real roots when
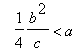
If c is negative, we can expect two real roots when
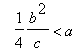
and no real roots when
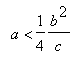
In this example, b = 5 and c = 4, so there are two real roots when a < 25/16 = 1.5625, one real root when a = 1.5625, and no real roots when a = 1.5625.
Consider again the equation
When we graph this equation in the xb plane with any particular value
of b, say b = 3, the intersection points of the curve in the xb
plane and the horizontal line correspond to the roots of the original equation
for that value of b. We have the following graph.
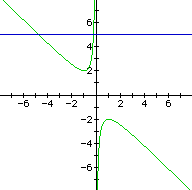
From the discussion above about the roots of the equation, we expect two real roots when
![]()
one real root when
![]()
and no real roots when
![]()
In this example, a = c = 1, so we expect two real roots when b
> 2 or b < -2, one real root when b = 2 or -2, and no real
roots when -2 < b < 2. From the graph, it is clear that we get two negative real roots of the original equation when
b
> 2, one negative real root when b = 2, no real roots for -2 < b < 2,
one positive real root when b = -2, and two positive real roots when b <
-2.
Notice that when a and c have opposite signs, 4ac
is always less than b2, so the equation will have two real
roots for all values of b.
As an example, let's consider the case when c = - 1 rather than + 1. Since a and c have opposite signs, we expect the equation to have two real roots for all values of b. When we graph the curve on the xb plane, we expect the curve to intersect every horizontal line at two distinct points. We see from the graph below that this is indeed the case.

In the following example the equation
is considered. If the equation is graphed in the xc plane, it is easy to see
that the curve will be a parabola. For each value of c considered, its graph
will be a line crossing the parabola in 0, 1, or 2 points -- the intersections
being at the roots of the original equation at that value of c. In the graph, the
graph of c = 1 is shown. The equation
will have two negative roots -- approximately -0.2 and -4.8.
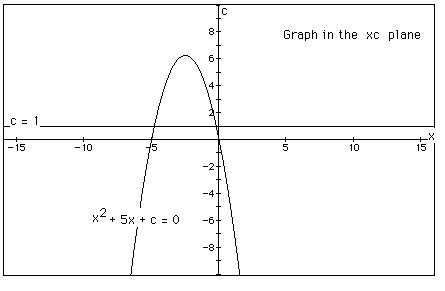
There is one value of c where the equation will have only 1 real root -- at c = 6.25. For c > 6.25 the equation will have no real roots and for c < 6.25 the equation will have two roots -- both negative for 0 < c < 6.25, one negative and one 0 when c = 0, and one negative and one positive when c < 0.
Does this agree with the previous discussion about the roots of the equation?
We expect the equation to have one real roots when
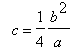
In this case, a = 1 and b = 5. We expect one real root when c = 6.25, which agrees with the graph.
When a is negative, we expect two real roots when
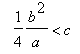
and no real roots when
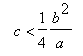
When a is positive, as in this example, we expect two real roots when
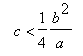
and no real roots when
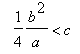
This agrees with the above conclusions from the graph.
Although it is common to find the roots to a quadratic equation using factoring or the quadratic formula, the roots can be found in other ways. In particular, graphing the equation
on the xa, xb, or xc plane and finding the intersection points of this curve with horizontal lines corresponding to values of a, b, or c, respectively, gives the roots of the original equation for those values of a, b, or c.