
This exploration will investigate the characteristics regarding the locus of points for different cases of the tangent circles disscussed on Dr. James Wilson's Assignment page: "http://jwilson.coe.uga.edu/EMT668/Asmt7/EMT668.Assign7.html"
In order to follow this exploration you may want to download the GSP script to create the different cases of tangent circles. To download the script you need to be able to run GSP 4.03 on your computer. To download the script, click here ->
The different cases for constructing the tangent circles are:
In both cases, the construction of the tangent circle is similar, only differring in the location of the segment j and k as shown in the sketch. The circle on the left is case b. and the circle on the right is case a.

A good question would be, how does this affect the placement of
the tangent circle?
After observing both cases, and trying to determine the relationship between the locus of points and the location of the smaller circle with respect to the tangent circle, I had found that the locus of points is primarily determined by the location of the tangent circle (shown in red on the above figures). When the tangent is interior to either circles then the locus of points constructs an ellipse, whereas when the tangent is exterior to either circles then the locus of points constructs an hyperbole.
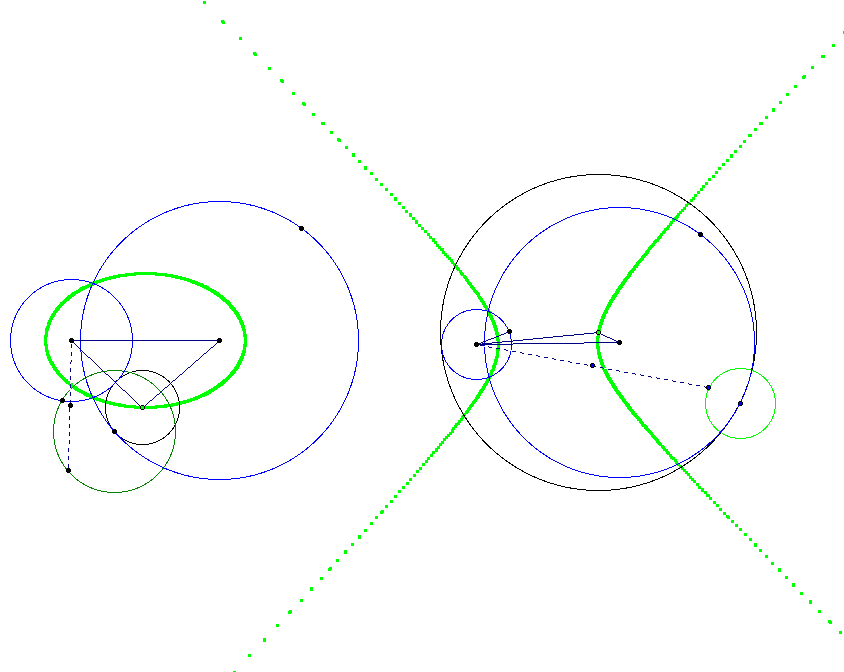
The light green collection of points represents the locus of points for each case. The left side represents the tangent circle interior to the parent circles, while the right side represents the tangent circle exterior to the parent circles.
This is a very problem rich environment of which I will only attempt to prove why the locus of points behave as either an ellipse or a hyperola.
Before I start the exploration, I will need to define ellipse and hyperbola:
Merrian Webster defines an ellipse as, "a closed plane curve generated by a point moving in such a way that the sums of its distances from two fixed points is a constant"
Merrian Webster defines a hyperbola as, "a plane curve generated by a point so moving that the difference of the distances from two fixed points is a constant."
I have highlighted fixed points, and constant in that they will be crucial to my attmept to prove that the locus of points for each case is indeed either a hyperbola or an ellipse.
Please refer to the figure below for my first attempt:

Since the Tangent circle (shown in red) is exterior to the parent circles, then we can claim that the locus of points (the trace of K as L follows circle D), will be a hyperbola. By the definition of a hyperbola, we need two fixed points and the difference of the points from the fixed distances is constant.
Triangle FDK is highlighted to better illustrate where the focii are and the relationship of the two fixed points (D,F). For this proof we want to show that points F and D are the fixed points are their distances will remain constant when point L is rotated about circle D. The goal is to show that DK- FK = R+r

Since the Tangent circle (shown in red) is interior to one of the parent circles, then we can claim that the locus of points (the trace of B as L follows circle D), will be an ellipse. By the definition of a ellipse, we need two fixed points and the sum of the points from the fixed distances is constant.
Triangle ABC is highlighted to better illustrate where the focii are and the relationship of the two fixed points (A,C). For this proof we want to show that points A and C are the fixed points are the sum of their distances will remain constant when point F is rotated about circle A. The goal is to show that AB+BC = R-r
For a GSP Sketch click here ->
Return to Assignment Matrix click here ->
Questions? E-mail: gt0353d@arches.uga.edu