The Richest Woman in Babylon

By: Denise Natasha Brewley

 

Did you know that if someone gave you a penny today, and you continued to double that amount each day for thirty days, you would be a millionaire by the end of the month?  Consider the following table constructed in MS Excel.  Each day is indicated and its respective amounts in dollars.  A scatter plot of the data generated is also given below.
Days
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
Amount in Dollars
$0.01
$0.02
$0.04
$0.08
$0.16
$0.32
$0.64
$1.28
$2.56
$5.12
$10.24
$20.48
$40.96
$81.92
$163.84
$327.68
$655.36
$1,310.72
$2,621.44
$5,242.88
$10,485.76
$20,971.52
$41,943.04
$83,886.08
$167,772.16
$335,544.32
$671,088.64
$1,342,177.28
$2,684,354.56
$5,368,709.12
 

We want to generate a function based on this data that will help us to project how much we can expect to have after the thirty day time period.  We will do this in two ways -- first we will solve this problem algebraically and then we will use MS Excel's function Wizard to verify our model.

It is important to note here that the behavior of our graph appears to be exponential.  An exponential function usually takes the form, y=a(xb).  Exponential functions are often used to represent money growth or population growth phenomena.  Since the amount is doubled each day, the data has a trend that helps us to find the equation of this exponential function.  Let b = 0 correspond to Day 1 and b = 3 correspond to Day 4.  Substituting the data provided and solving gives us the exponential function,

y = (.01)2b, for b > 0

But let's say that we did not know how to write the function for the given data.  We will now use the tools in MS Excel to help figure out what type of trend line best fits our data.  If we use the Chart Wizard, there is a Format Trend Line option.  Since our data appears to be exponential, we can select the exponential trendline type.  With the data that we plotted, we can now add a trend line as indicated below in red.  

 

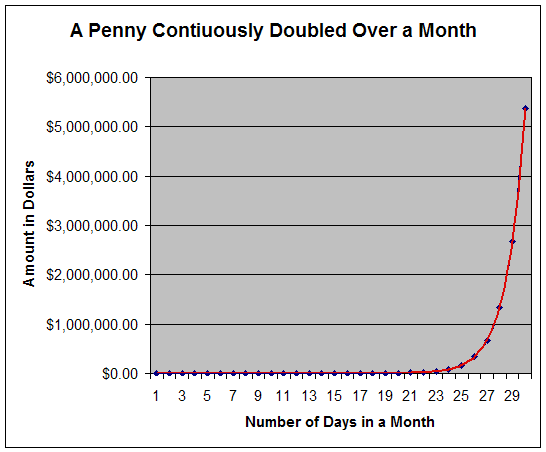
So now we can figure out when the richest woman in Babylon will become a billionaire.  Can you figure out on what Day she will become a billionaire based on the model we previously found?  CLICK HERE for the solution.

BACK TO MY HOMEPAGE