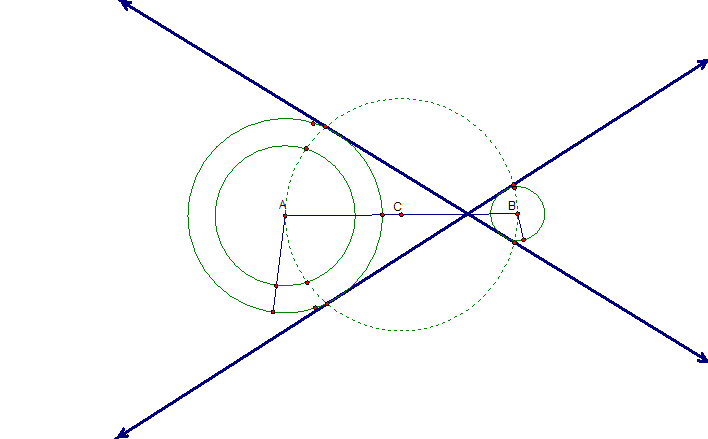
Posed Problem:  Construct the common tangents to two given circles. 

To begin this construction, we first draw two circles and create a segment through the two centers circles, A and B. The radius of each circle is also indicated.

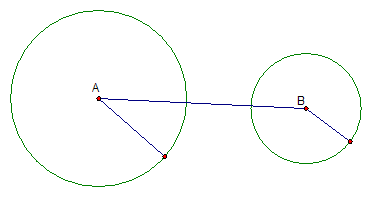
We then create a circle whose center is a point on circle A, but whose radius is that of circle B.

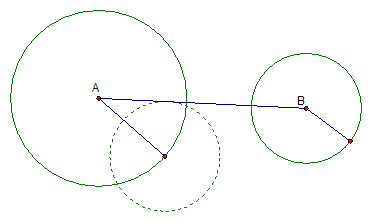
In circle A, the radius that is left over, we construct another circle whose center is also A.  We then hide the dashed circle.

We first find the midpoint of line segment AB.  Then, Circle C is now constructed and its diameter is the centers of Circle A and Circle B.

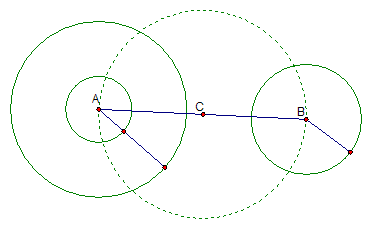
The intersection of a point on little Circle A and Circle C is found. A ray through this intersection point is also found.

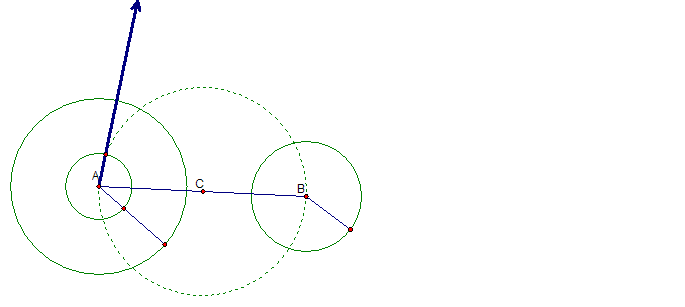
We then find the perpendicular bisector of the ray.  Finally, the tangent line to these two circles can be observed.

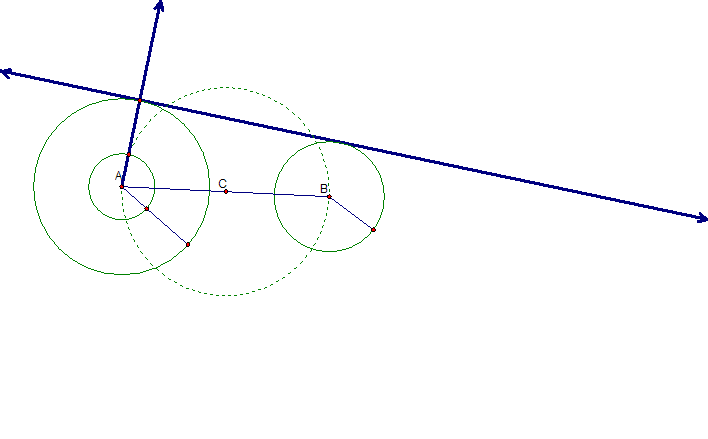
We can extend this construction by reflecting the tangent line constructed over line segment AB.

There is second set of tangent lines that we found.  I took the intersection point of the top of the circle A with circle C and the intersection point of the bottom of circle C with circle B.  I then constructed a line segment.  I repeated the steps, only this time it was the bottom of circle A and circle C and the top of circle C and circle B.

BACK TO MY HOMEPAGE