Predicting the Cost of Stamps

by

Eileen Murray

In Assignment 12, I investigated the price of stamps using data from 1933 through 1995. For this section, I will be extending my data to 2006 because this is the most current data that is available. I will try to determine if my predictions and functions change with this new data.

Year

Cost in Cents

1933

3

1958

4

1963

5

1968

6

1971

8

1974

10

1976

13

1978

15

1981

18

1982

20

1985

22

1988

25

1991

29

1995

32

1999

33

2001

34

2002

37

2006

39

First I entered this information in an EXCEL spreadsheet and used the Chart and Trendline commands to come up with prediction functions. I used the data from 1933 to 2006 as well as 1958 to 2006. I left out the 1933 since that point was further removed from the rest of the data and was therefore skewing the functions. I had the computer determine the R^2 values as well to help me determine which function was the best predictor.

As it turned out, the data without 1958 consistently gave me more accurate predication functions, hence those were the functions on which I focused. Below I have reproduced the linear, quadratic and linear predication functions, but to see all of my analysis, go to my EXCEL file.

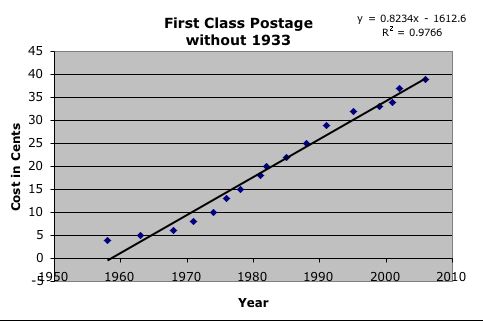
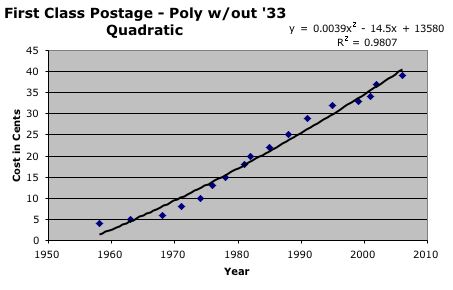
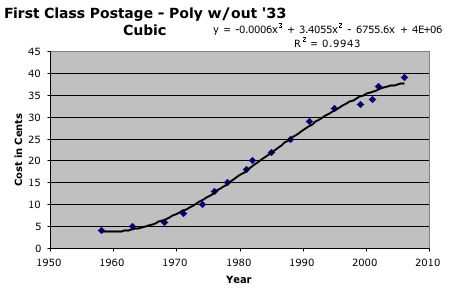
In this investigaionEven though the cubic function has the highest R^2 value, this is not a good prediction function because it will start to decrease in 2008. Therefore, I narrowed my choice down to the linear and quadratic functions. Below is a table comparing these two functions.

 Type

Equation

R^2

$0.74

 $1.00

Linear from 1958

 y = 0.8234x - 1612.6

0.9766

2048

 2079

Quadratic from 1958

 y = 0.0039x^2 - 14.5x + 13580

 0.9807

 2036

 2055

It seems from this crude analysis that the main difference between these two funtions is how they will predict the distant future. At first the functions look almost identical, but after about 2014, the functions start to follow very different patterns.

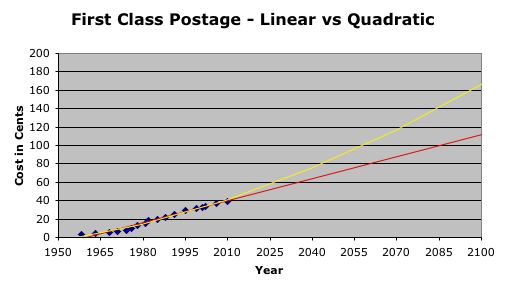
One question to ask that may make the decision a bit easier is for us to try to determine when the next increase is expected based on these models. Now, since these are both continuous functions, this will not be exact. We have to account for the fact that the stamps will never be a fraction of a cent, nor will they likely be changed in the middle of the year. Therefore, I searched for th first time a integral year matched with an integral cent value. This proved difficult. Since the functions were predictions, not even 2006 was giving me an integral cent value. Therefore, I needed to make a decision.

Based on the historical data, it looked like the next increase would be between 1 and 4 cents. Thus, I generated a table of values to determine when each function got closest to this range.

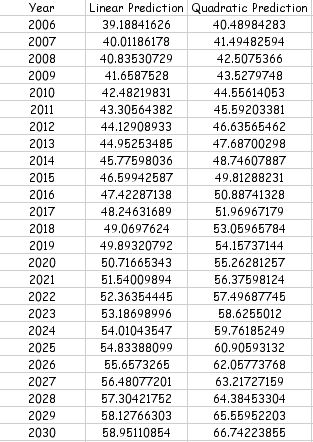
From this table, it seems that the quadratic function would be increasing the cost by at least one cent every year. The linear function predicts a slightly more modest increase of one cent every two years. I would like to think that the linear function is the better predictor, but that is just my pocketbook talking!

As far as the analysis of stamp data historically doubling every year, I conclude that the person who presented this theory must have only had data from 1968 to 1988. Beyond this, the doubling pattern seems to be way off of the real data as well as far removed from the two prediction functions computed.

 

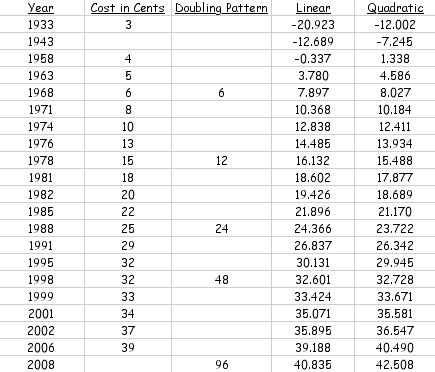
In the end, to determine which model is the best, we most likely have to wait and see what good ol' Uncle Sam does with the price of postage.

 

Return to Final Assignment page.