

Problem: Given two circles and a point on one of the circles. Construct a circle tangent to the two circles with one point of tangency being the designated point.
First, we will begin with a large circle and a smaller circle inside the large circle as shown below.

We will explore the tangent circle to these two circles by keeping the smaller circle external to the tangent circle and the tangent circle internal to the large circle. The directions for the construction in Geometer's Sketch Pad are in the Assignment instructions.
Here is the resulting product for the tangent circle of two given circles. When exploring, notice the path of the center of the tangent circle as it moves around the smaller circle. The red circle is the circle tangent to both of the given circles. Click the link below to start your exploration.
As you can see from GSP, the locus of the center of the tangent circle creates an ellipse. The foci of the ellipse are the center of the two original circles. Did you have any other observations? Below are a few observations.
Extension: The blue dotted line in the link below is always tangent to the ellipse we explored above. The line is part of our original construction. Click below to see the tangency lines.
Secondly, we will look at the tangent circle to two intersecting circles as shown below.
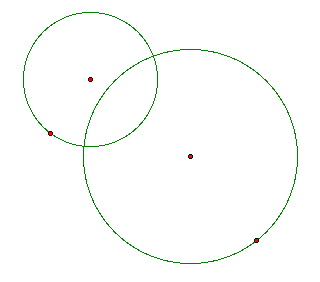
The construction is the same as our first construction, with the exception of moving the circles to be intersecting. You can explore below. Notice the locus of the center of the tangent circle.
The locus of the center of the tangent circle formed an ellipse again. Did you observe that the ellipse intersects with the two points of intersection of the original two circles? The foci were still the centers of the two original circles. Did you also notice that the tangent circle is internal to the larger circle and then becomes internal to the smaller circle? Below are more observations.
Thirdly, we will look at the tangent circle (if it exists) to circles that are disjoint as shown below.
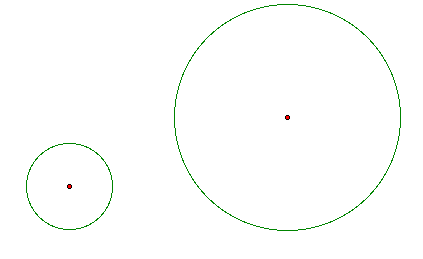
The construction is the same as our first construction, with the exception of the circles being disjoint. You can explore below. Notice the locus of the center of the tangent circle when it exists.
Did you notice what path the locus of the center of the tangent circle took? You should have noticed that it formed a hyperbola. Also, notice that there was not always a tangent circle. The radius of the tangent circle increased rapidly when the circle got close to becoming the tangent line between the two circles. At one point, the "tangent circle" becomes a tangent line. This is why the locus of the center of the tangent circle is a hyperbola. The asymptotes are where the tangent circle becomes the tangent line to both of the original circles.
Also, notice that as the original circles get closer in size, the two sections of the hyperbola get farther apart. Did you have any other observations?