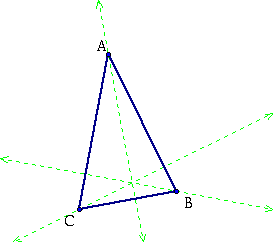
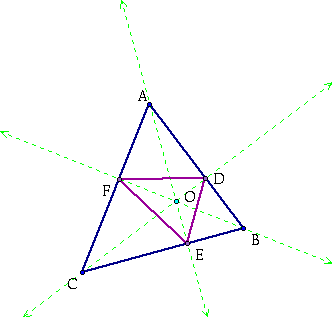
We first must draw the perpendicular bisectors (altitudes) of triangle ABC
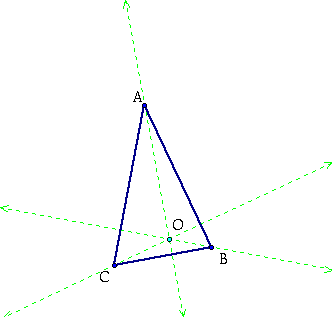
Next where the three altitudes connect, forms the orthocenter, labeled O.
Now we can go ahead and label the points at which the altitudes cross the sides of triangle ABC, we will label these points D, E, and F.
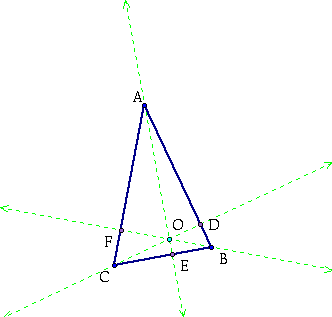
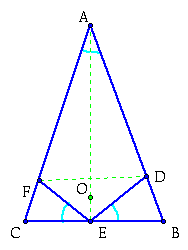
Now points D, E, and F, will form a triangle:
Now what we did to prove, is that the
FEC is equal to DEB, and that both of these
angles are equal to CAB. We are going to do this by looking
at quadrilateral ODBE.
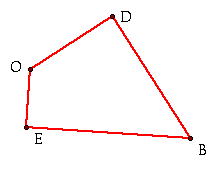
Since this quadrilateral is inscribed
in the triangle and formed by the perpendiculars, we know that
E and D, are right angles.
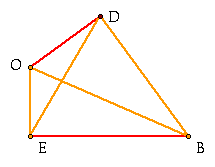
Additionally looking back, if we connect
DE and OB, we see that DBO and DEO (in orange) both share
arc OD.
Therefore, DBO = DEO (ABF = DEA). Now look
at triangle ABF.
We know that triangle ABF is a right triangle,
since F is one of the perpendiculars and therefore a right angle.
Due to this fact we know that A and B must therefore
add to 90 degrees (A and B are complementary).
Now look at the figure below:
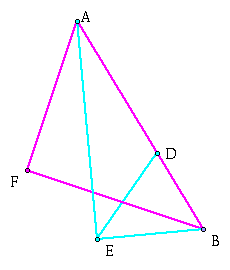
We additionally can see that since E
forms a right angle, we know that BED must be complementary
with DEA. Now since above we showed that ABF (DBF) was equal
to DEA (DEO), we must conclude that DEB = A. This is the
first part of what we needed to show.
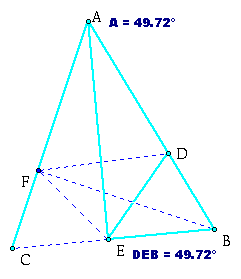
Now we are half way done. Using this same
process we can now look at quadrilateral FOEC. In doing this we
will see that A = FEC, as shown below.
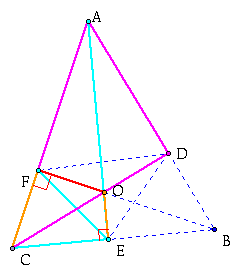
This figure is a combination of all the steps above. Click here to see a progression of these steps. Following these through will give us
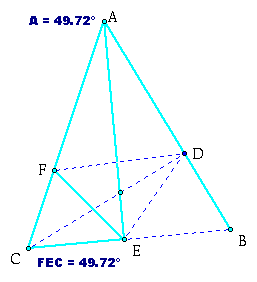
So now we know that A = FEC = DEB.
