
Materials:
Paper triangle, sheet of paper, pencil, protractor, ruler
Construction:
1.
Pick one side of your triangle. Fold
that side in half to find its midpoint.
2.
Trace the triangle onto your sheet of paper.
Think carefully about where you want to put the triangle on your paper to
trace it.
3. Rotate the triangle 180 degrees about the midpoint that you found.
5.
Label the vertices of your figure with the letters A, B, C, and D as in
the figure below. Also label the
point that you rotated about with the letter X.

Questions
1.
What kind of figure have you created?
____________________
2. How are the two triangles related?
The
two triangles are _______________.
Opposite
Sides of a Parallelogram
3. Use your ruler to measure sides AB and CD.
Length of AB __________
Length
of CD __________
4. Use your ruler to measure sides BC and DA.
Length of BC __________
Length
of DA __________
5.
Looking at
your answers to numbers 4 and 5, complete the following theorem.
The
opposite sides of a
parallelogram are _______________.
Opposite
Angles of a Parallelogram
6.
Use your protractor to measure ÐBAD
and ÐDCB.
Measure
of ÐBAD __________
Measure of ÐDCB __________
7.
Use your protractor to measure ÐADC
and ÐCBA.
Measure
of ÐADC __________
Measure
of ÐCBA __________
8. Looking at your answers for numbers 7 and 8, complete the following theorem.
Opposite
angles of a parallelogram are _______________.
Consecutive
Angles of a Parallelogram
9. Looking at numbers 7 and 8, calculate the following:
ÐBAD + ÐADC = __________
ÐADC + ÐDCB = __________
ÐDCB + ÐCBA = __________
ÐCBA + ÐBAD = __________
Looking at your results, complete the following theorem.
The
consecutive angles of a parallelogram are _______________.
Diagonals
of a Parallelogram
10. Draw the diagonal AC. (Use your ruler!!) Your figure should now look something like the following:
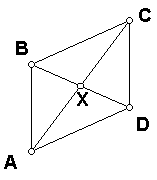
11. Measure the segments AX and CX.
Length of AX __________
Length
of CX __________
12. Measure the segments BX and DX.
Length of BX __________
Length
of DX __________
Looking at your answers for numbers 12 and 13, complete the following theorem.
The
diagonals of a parallelogram _______________ each other.