

![]()
Case 3:
![]()
As an example, let's consider the equation
![]()
The graph of this equation is the following:
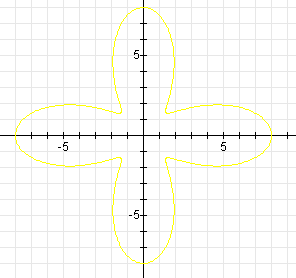
We see that this graph has k = 4 leaves, and the graph does not go all the way to the origin. The points farthest from the origin are | a | + | b | = 8 units from the origin, and the points closest to the origin are | a | - | b | = 2 units from the origin.
As before, when a and b are both negative, when k
is odd, the graph is rotated
![]() /k radians from the
case when a and b are both positive. When k is even,
the graph is exactly the same as the case when a and b are both
positive. Here are some examples:
/k radians from the
case when a and b are both positive. When k is even,
the graph is exactly the same as the case when a and b are both
positive. Here are some examples:
![]()
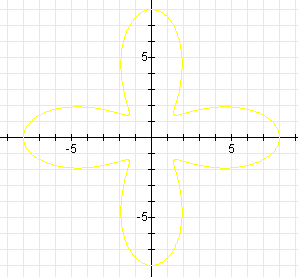
This graph looks the same as the above graph of
![]()
Let's look at

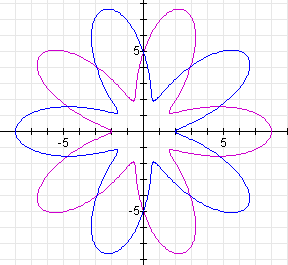
Also as before, when a is negative and b is
positive, when k is odd, the graph is the same as the case when a
and b are both positive. When k is even, the graph is
exactly the same as the case when a and b are both positive, but
it is rotated
![]() /k radians.
Let's look at an example where a is negative, b is positive, and k
is even.
/k radians.
Let's look at an example where a is negative, b is positive, and k
is even.
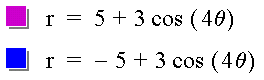
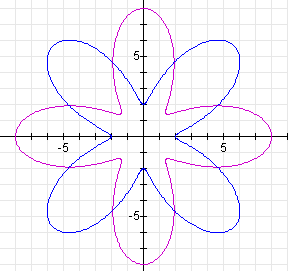
Also as before, when a is positive and b is
negative, he graph is the graph of the case when a and b are both
positive, but it is rotated
![]() /k radians,
regardless of whether k is odd or even.
/k radians,
regardless of whether k is odd or even.

Conclusions about the graph of the polar equation
![]()
when
![]()
The rose has k leaves, equally spaced about the origin.
The length of each leaf (the distance from the origin to the point farthest from the origin) is | a | + | b |.
The distance from the origin to the points closest to the origin is | a | - | b |.
When a and b are both positive, the end of one leaf is on the positive x-axis.
When a and b are both negative and k is
odd, the graph is the graph when a and b are both positive rotated
![]() /k radians.
/k radians.
When a and b are both negative and k is even, the graph is the same as the graph when a and b are both positive.
When a is negative, b is positive, and k is odd, the graph is the same as the graph when a and b are both positive.
When a is negative, b is positive, and k is
even, the graph is the graph when a and b are both positive
rotated ![]() /k radians.
/k radians.
When a is positive, b is negative, and k is
odd, the graph is the graph when a and b are both positive rotated
![]() /k radians.
/k radians.
When a is positive, b is negative, and k is
even, the graph is the graph when a and b are both positive
rotated ![]() /k radians.
/k radians.