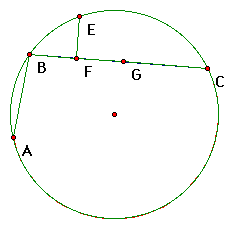
Again, recall our original diagram.

Now, that we have proven the Theorem of the Broken Chord, where does this lead?
What if the measure of minor arc EC is 2x and minor arc BE is 2y.
First, we need to show that the length of the chord EC would be 2 sin x. So, start by constructing the triangle EHC with H the center of the triangle.
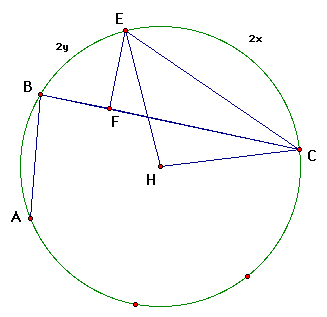
Now, angle EHC is 2x because the measure of an angle with a vertex at the center of the circle is equal to the intercepted arc measure. Triangle EHC is iscoceles because HC and HE and both radii of the circle with a measure of 1 since this is a unit circle.
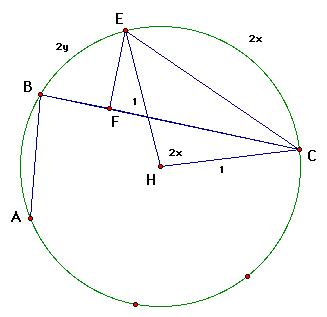
Since this is an isosceles triangle, the bisector of angle EHC is also the perpendicular bisector of EC. So, constuct this bisector and find the point of intersection with side EC and call it I.

We can now prove triangle EHI congruent to triangle CHI by SAS. First, we have already shown that HC is congruent to HE because they are both a radius of a circle. Second, ray HI is the angle bisector of angle EHC, angles EHI and CHI must be congruent. Finally, Since segment HI is in both triangles it is congruent to itself by the reflexive property. Therefore, triangle EHI is congruent to triangle CHI by SAS.
This is important for two reasons. First, this allows us to find the values of angles EHI and CHI to both be x. Second, segments EI and CI are now congruent by CPCTC.
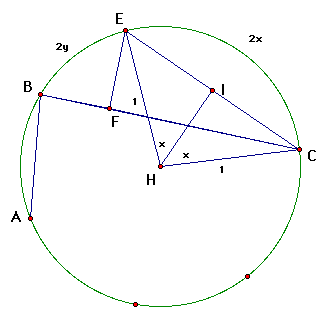
Since we have right triangles because HI is the perpendicular bisector of EC, the side opposite an angle of a right triangle is equal to the sin of the angle. That tells us that since the measure of angle IHC is x that the length of segment IC is sin x and since the measure of angle EHI is x that the length of segment EI is sin x.
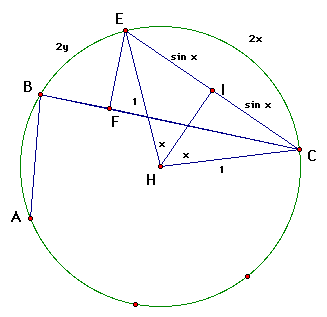
Thus, a simple addition allows us to show that the length of EC equals sin x plus sin x which is 2 sin x.
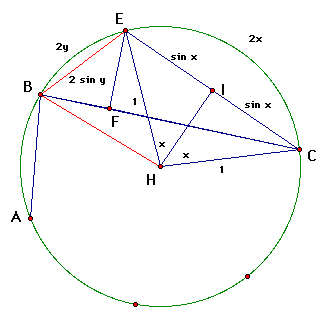
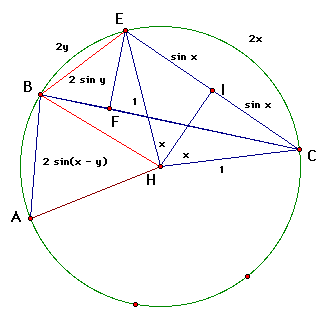
The next segment that we are interested in is AB, one of the segments of the broken chord. Recall that E is the midpoint of arc AEC. Thus, minor arc AE is equal to minor arc EC which has a length of 2x. That means that minor arc AB is equal to minor arc AE minus minor arc BE or 2x - 2y. Now, construct segment AH and we are able to apply the theorem from above again. That means that the length of AB is 2 sin x - 2 sin y which is 2 (sin x - sin y) or 2 sin (x - y).
We can now find the length of segment FC, another segment of the broken chord. First, note that angle ECB intersects minor arc EB which has length of 2y. This tells us that the measure of angle ECB is half of that or y. Now, we use our right triangle EFC and our trig functions to find the length of FC.
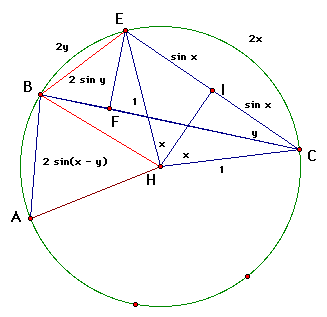
Then cos y equals FC divided by 2 sin x. Solve this for FC and find that FC equals 2 sin x cos y.
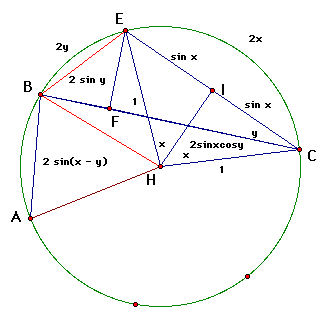
We can now find the length of segment FB, the final segment of the broken chord. First, note that angle EBC intersects minor arc EC which has length of 2x. This tells us that the measure of angle EBC is half of that or x. Now, we use our right triangle EFB and our trig functions to find the length of FB.
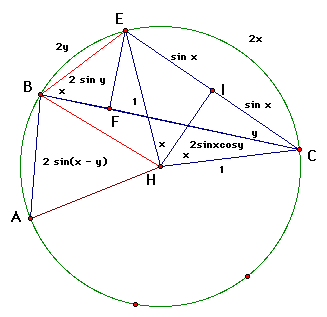
Then, cos x equals FB divided by 2 sin y. Solve for FB and find that it is 2 sin y cos x.

This now leads us to some trig identities that we all know and love. Click on the links below to see how the theorem of the broken chord can be used to prove two common trig identities.
sin(x-y) = sinxcosy - sinycosx
sin (x+y) = sinxcosy + sinycosx
Return to the Broken Chord Main Page