Parametric Functions
By Vilma Mesa
Abstract
In this paper I want to present an analysis of the following parametric
function:

Different cases were considered by changing the constants a, k, h, and
j, of this general form. The use of the tecnology showed to be very helpful
for understanding the behavior of the function in those cases and to find
patterns of behavior that were later verified analitically. The problem
may help students to understand the role of the parameter t in representations
as the given above, and also the meaning of points that belong or that does
not belong to a graph.
Problem
Parametric functions is a topic that presents some difficulties for students.
The need to differentiate the role of a parameter that takes some values
from a fixed interval, together with the fact that when the parameter exhausts
the interval the student gets a graph whose domain is the whole line or
that does not seem to behave as a function (because of the ''vertical line"
test) are difficult to accomplish even after long practices. One of the
main difficulties is the burden of computation for drawing the graphs. The
use of technology can alleviate that burden and also give the students a
space for exploring parametric functions from different perspectives. The
following problem was developed having in mind this two needs. The exploration
took place as a modification of the following problem:
Produce the graphs of the function parametrized as:

Interpret and analyze what is worth to change to explore and
understand the graphs.
What follows is the solution I produced acting as a student that uses
by the first time a specialized software for graphing parametric functions
(Theorist). The experienced teacher will be able to recognize the possible
shortcomings and advantages of the approach followed, and also use the ides
presented for suggesting further explorations.
Solution
First of all let's obtain the graphs of this function:
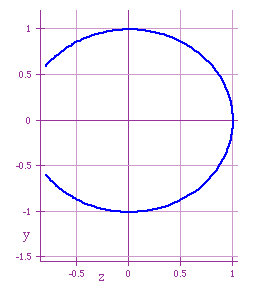
Several explorations can be done changing the symbolic expression of
this function. For example:
Case 1: Preserve the general form, and change the constants,
1 and 2 to different values
Case 2: Preserve the functions in the denominator and change the functions
in the numerator (for example other polynomial functions or other type of
functions)
Case 3: Preserve the functions in the numerator and change the functions
in the denominator
This exploration will show the results for Case 1 only.
Case 1: Changing the Constants
Consider the function defined by

In this expressions we can either change a, k, h, or j. I considered
each case separately.
Changing a Changing k h=j h = -j
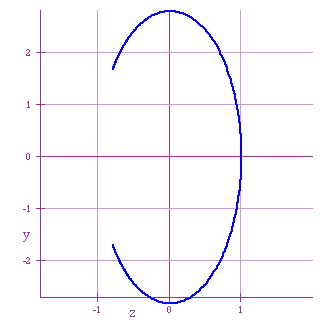

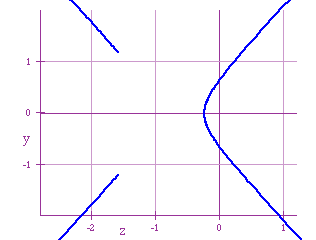
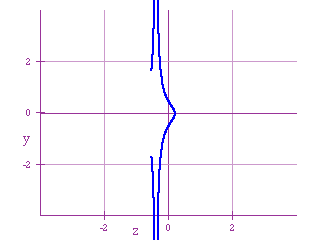
Conclusions
The use of technology certainly helped me to explore this problem. Although
I do not consider that it was completely analyzed, the cases studied gave
a broad view of the behavior of the function, and they suggest other possible
extensions of the problem. The use of Theorist allowed to produce animations
and to interact with the function. The use of other tool like a spreadsheet
probably can highlight other aspects about the relation between the change
of t and the change of the other parameters.
Back to my Home Page








