Ken Montgomery

EMAT 6690

INSTRUCTIONAL UNIT: Distance, Area, and Volume

Surface Area

The three-dimensional analog of perimeter is surface area. Just as perimeter is the one-dimensional measure or length of the boundary of a two-dimensional area, surface area is the two-dimensional measure or area of the boundary of a three-dimensional space. To find surface area, we generally find the sum of the areas of the individual surfaces, covering the bounded, three-dimensional space. Recall that in finding perimeter, we found the sum of the lengths of the individual sides, surrounding the two-dimensional area. To investigate surface area, we will examine nets or two-dimensional representations of the surfaces of three-dimensional objects.

Cube

A cube is a prism, or three-dimensional object with flat surfaces and with all three dimensions (length, width and height) equal. The length of one edge of the cube, s, in Figure 1, thus represents the lengths of all edges.

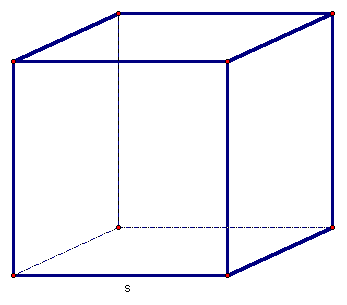
Figure 1: A cube, with side length, s

The net for a cube is given in Figure 2.

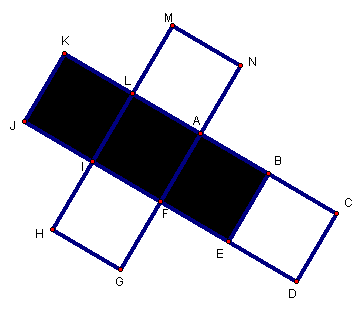
Figure 2: Net of a cube

The surface area of a cube is equal to the sum of the individual areas of its surfaces. The six squares, which comprise the total surface area of the cube, are congruent. The formula for the surface area of the cube is therefore given in Equation 1.

Equation 1:

Rectangular Prism

A rectangular prism is different from a cube, in that the three dimensions (length, width and height) are not necessarily equal (Figure 3).

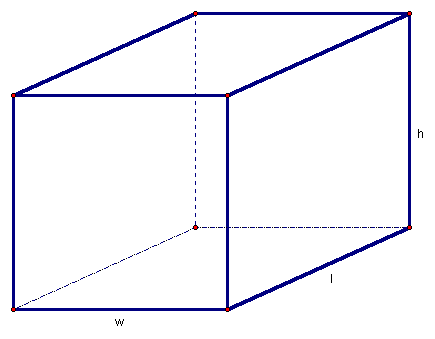
Figure 3: A rectangular prism with dimensions, length, width and height

The net for a rectangular prism is given in Figure 4.

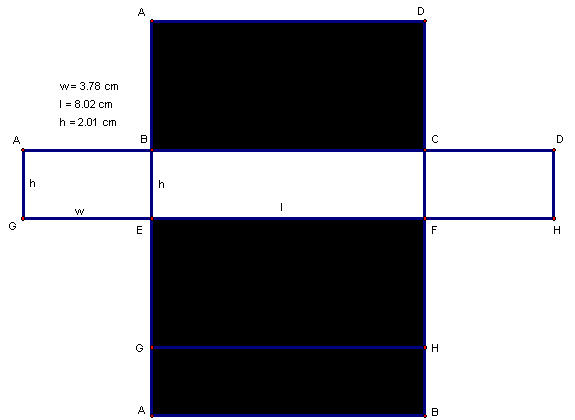
Figure 4: Net for a rectangular prism

The surface area of the rectangular prism, like the surface area of the cube, is equal to the sum of the areas of the individual surfaces. The total surface area is therefore, equal to the sum of areas of the front, back, top, bottom, left side and right side (Equation 2).

Equation 2:

In Figure 4, we have the net for a particular rectangular prism. Notice that the rectangle representing the front is congruent to the back (), the top is congruent to the bottom (), and the left side is congruent to the right side (). Substitution from this system of congruencies, we obtain Equation 3.

Equation 3:

Combining like terms in Equation 3 yields Equation 4.

Equation 4:

Each of these areas is a rectangle, so we must apply the area formula for the rectangle (Equation 5) in our calculation of surface area.

Equation 5:

The rectangular prism has three dimensions: length, width and height. We substitute the appropriate dimension into the rectangle area formula to obtain the areas for the front, top and left side (Equations 6, 7 and 8).

Equation 6:

Equation 7:

Equation 8:

Substituting Equations 6, 7 and 8 into Equation 4 yields Equation 9.

Equation 9:

Factoring out a 2 yields Equation 10.

Equation 10:

Pyramid

The net for a square pyramid is shown in Figure 5.

Figure 5: Net of a Square Pyramid

Pyramids may have any polygon as a base. The pyramid in Figure 5 has a square base and is thus referred to as a Square Pyramid. To find the surface area of a pyramid, we find the sum of the areas of the individual surfaces. Since there are four congruent sides to the base, there are four triangles, the vertices of which are the common point E and which therefore have congruent heights. We refer to the height of any one of these triangles as the slant height, l, since this is not the height of the pyramid, but rather the height of a slanted triangle. The area for a representative triangle is thus given in Equation 11 where b, the base of the triangle is one side of the base, B.

Equation 11:

For a pyramid with a general base of any regular polygon, the surface area is equal to the sum of the individual areas of the n, triangles and the surface area of the base (Equation 12), where n is the number of sides of the base. For the square, pyramid in Figure 5, we have n = 4 and the base, B, would be calculated using the formula for the area of a square.

Equation 12:

We rearrange the second term of the equation, by applying the commutative property of multiplication to obtain Equation 13.

Equation 13:

Notice the product of the side-length (b) and the number of sides (b) is equal to the perimeter (P) of the base, which with the area of the base (B), in the first term gives the general surface area formula for a pyramid (Equation 14).

Equation 14:

Find the surface area of the hexagonal pyramid in Figure 6.

Figure 6: Hexagonal Pyramid

In Figure 6, the side-length is , and the slant height is . Since the base is a regular polygon, the calculated surface area of the pyramid is given in Equation 15.

Equation 15:

Cone

To find the surface area of the cone, we find the sum of the areas of the surfaces in the net, shown in Figure 7.

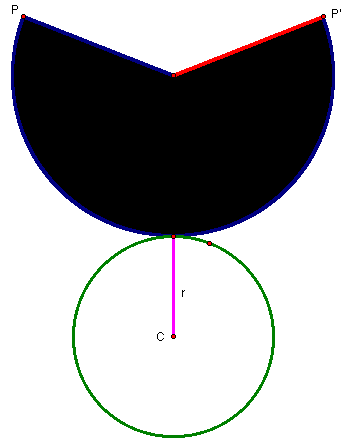
Figure 7: Net of a Cone

Notice in Figure 7, that the arc length of the sector must be equal to the circumference of the base. To find the area of the base, we need only apply the formula for the area of a circle (Equation 16).

Equation 16:

However, we must also find the area of the sector and we do not know the measure of . We will, therefore, set up a proportion between sector area and total circle area and arc length and total circumference (Equation 17).

Equation 17:

We know that the arc-length of the sector is equal to the circumference of the base; and we substitute l (the radius of the sector) into the formulas for area and circumference for the circle corresponding to the sector (Equation 18).

Equation 18:

We then solve Equation 18 for the sector area (Equation 19).

Equation 19:

Simplifying Equation 19, yields the area of the sector in terms of r (radius of the base) and l (radius of the sector) in Equation 20.

Equation 20:

To find the total surface area of the cone, we thus add the areas of the base and sector (Equation 21).

Equation 21:

Simplifying via the distributive property yields the formula for surface area yields Equation 22.

Equation 22:

Cylinder

The net (2-dimensional representation of a cylinder) is given in Figure 8.

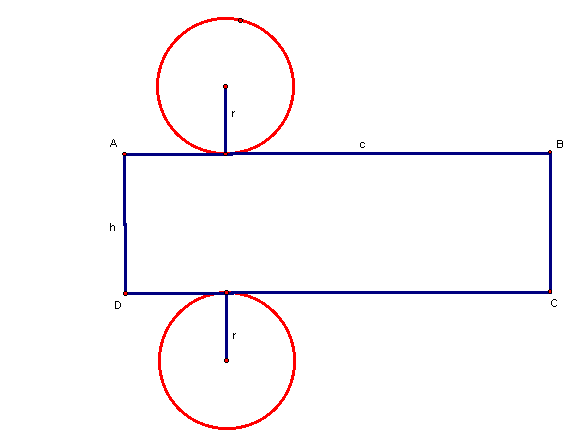
Figure 8: Net of a Cylinder

Here, we see that the total surface area of the cylinder is equal to the sum of the two circles (the top and bottom) and the rectangle (the side area of the cylinder). The area of the circle is given in Equation 23.

Equation 23:

Since the circles are congruent, they have the same area and the combined area of the two circles is given in Equation 24.

Equation 24:

The length (l) of the rectangle is equal to the circumference of the circles and the width is equal to the height (h). The area of the rectangle is thus given in Equation 25.

Equation 25:

The sum of the areas of the individual surfaces, thus give the total surface area of the cylinder (Equation 26).

Equation 26:

Simplifying, via the distributive property yields the formula for the surface area of a cylinder (Equation 27).

Equation 27:

Sphere

Figure 9: Sphere with radius, r

The formula for the surface area of a sphere is given in Equation 28 and can be derived using integral calculus.

Equation 28:

However, one may conceptually understand the surface area as the measure of a “fabric” which will cover the sphere. The rectangular measurement would be given by the area formula for a rectangle (Equation 29).

Equation 29:

The length and width would be the circumference and diameter, respectively yielding Equation 30.

Equation 30:

The formulas for circumference and diameter are given in Equations 31 and 32, respectively.

Equation 31:

Equation 32:

Now substituting from Equations 31 and 32 into Equation 30 gives us Equation 33.

Equation 33:

Simplifying Equation 33 results in the formula for the surface area of a sphere (Equation 28 and Equation 34).

Equation 34:

Return to EMAT 6690